Progress was made for the first time in decades, apprentices Tao Zhexuan and Zhao Yufei broke through combinatorial mathematics problems

Recently, for the first time, progress has been made on a decades-old unsolved mathematical problem.

Driving this progress are UCLA graduate student James Leng and MIT mathematics graduate student Ashwin Sah, and Columbia University assistant professor Mehtaab Sawhney. Among them, James Leng studied under the famous mathematician Terence Tao, and Ashwin Sah studied under the discrete mathematics master Zhao Yufei.

Paper address: https://arxiv.org/pdf/2402.17995

To understand the breakthrough achieved in this research, you need to start with arithmetic progressions.

The sum of the first n terms of an arithmetic sequence is called an arithmetic series, also called an arithmetic series. In 1936, mathematicians Paul Erdős and Pál Turán conjectured that if a set consists of nonzero fractions of integers (even 0.00000001%), then it must contain an arbitrarily long arithmetic series. The only sets that can avoid arithmetic series are those that contain a "negligible" part of the integers. For example, the set {2, 4, 8, 16, …}, where each number is twice the previous number, is spread out along the number axis without progression.

In 1975, mathematician Endre Szemerédi proved this conjecture. His work gave rise to a variety of research directions that mathematicians are still exploring today.

Mathematicians established Szemerédi’s result in the context of a finite set of numbers (all integers from 1 to some number N). How much of the initial pool can be used in the set before inevitably including a forbidden series? How does this proportion change as N changes?

For example, let N be 20, how many of these 20 numbers can be written down while still avoiding series that are 5 or more numbers in length? The answer, it turns out, is 16% to 80% of the initial pool.

Szemerédi was the first to show that as N grows, this fraction must shrink to zero, and mathematicians have since tried to quantify how quickly this happens.

Last year, groundbreaking work by two computer scientists nearly solved the problem of three-term series, such as {6, 11, 16}. But the problem becomes more difficult when you try to avoid arithmetic series of four or more terms. This is because longer series reflect underlying structures that are difficult to reveal using classical mathematical methods.

The numbers x, y and z in the three-term arithmetic series always satisfy the simple equation x – 2y + z = 0 (taking the series {10, 20, 30} as an example: 10 – 2*(20) + 30 = 0), it is relatively easy to prove whether a set contains numbers that satisfy this condition. While the numbers in a four-term series must also satisfy the more complex equation x^2 – 3y^2 + 3z^2 – w^2 = 0, a series with five or more terms must satisfy an even more complex equation. This means that sets containing such series will exhibit more subtle patterns. It would also be harder for mathematicians to prove whether such a pattern exists.

In the late 1990s, mathematician Timothy Gowers proposed a theory to overcome this obstacle. He was later awarded the Fields Medal, mathematics' highest honor, in part for this work. In 2001, he applied his method to Szemerédi's theorem, proving better bounds on the maximum set size, avoiding arithmetic series for any given length.

In 2022, James Leng, then a second-year graduate student at UCLA, began to understand Gowers’ theory. He did not consider Szemerédi's theorem. Instead, he hopes to answer questions about Gowers' approach.

However, after trying hard to explore for more than a year, he found nothing.

Sah and Sawhney, who have been thinking about related issues, learned about Leng’s work and were very interested. Sawhney even said: "I am surprised that I can think like this."

Sah and Sawhney realized that Leng's research might help them make further progress on Szemerédi's theorem. Within a few months, three young mathematicians figured out how to get better upper bounds on the size of sets without pentterm series. They then extended their work to series of arbitrary lengths, marking the first progress on the problem in the 23 years since Gowers' proof.

Let 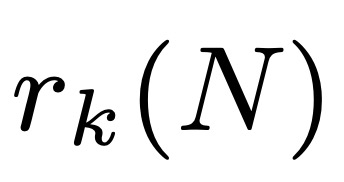denote 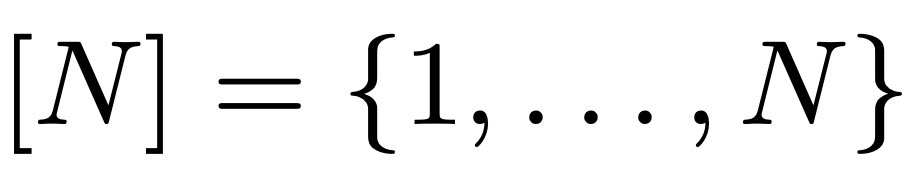, the size of the largest subset of an arithmetic series without k terms. Leng, Sah and Sawhney showed that for k ≥ 5, there exists c_k > 0 such that .

Research Team

The first author of the paper James Leng is a graduate student in mathematics at the University of California, Los Angeles (UCLA), and received his undergraduate degree from the University of California, Berkeley. He studied under the famous mathematician Terence Tao.

James Leng’s research interests include arithmetic combinatorics, dynamical systems, Fourier analysis, etc. His research has also been supported by an NSF graduate fellowship.

                                                                                 ^{. In the summer of 2016, 16-year-old Sah won the gold medal in the International Mathematics Olympiad (IMO). The following year he entered MIT to study.}

                                                                            Ashwin Sah

^{During his studies at MIT, there were two people who played an important role in Sah’s mathematical development. The first one is Professor Yufei Zhao, a master of discrete mathematics, who is also Sah’s graduate tutor.} The second one is Mehtaab Sawhney, they met in class and became friends. Later, the two did research together and discussed various topics in the field of discrete mathematics, such as graph theory, probability theory and the properties of random matrices. Ashwin Sah and Mehtaab Sawhney met at the end of 2017 when they were undergraduate students at (MIT). Since then, the two have written an incredible 57 mathematical proofs together, many of which have had profound consequences in various fields.

                                                                                                                                                                            Mehtaab Sawhney

^{Mehtaab Sawhney is currently an assistant professor at Columbia University. His research interests include combinatorics, probability, and theoretical computer science, among others.}

Reference link: https://www.quantamagazine.org/grad-students-find-inevitable-patterns-in-big-sets-of-numbers-20240805/

The above is the detailed content of Progress was made for the first time in decades, apprentices Tao Zhexuan and Zhao Yufei broke through combinatorial mathematics problems. For more information, please follow other related articles on the PHP Chinese website!