Home >Backend Development >Python Tutorial >How to use Python to implement genetic algorithm to solve the Traveling Salesman Problem (TSP)?
How to use Python to implement genetic algorithm to solve the Traveling Salesman Problem (TSP)?
- WBOYforward
- 2023-05-08 19:46:182610browse
TSP problem
So before we start, let’s describe this TSP problem in detail. Friends who have done digital modeling, or have been exposed to intelligent optimization or machine learning should all know this. Of course, for the sake of the universal audience of this article, we will try our best to make it as perfect and clear as possible here, so that we can actually solve the problem.
So the problem is actually simple, it goes like this:
In our N-dimensional plane, today we use this two-dimensional plane Come on, there are many cities on this plane, and the cities are connected to each other. Now we need to find the shortest path to visit all the cities. For example we have cities A, B, C, D, E. Now that we know the coordinates between cities, which is equivalent to knowing the distance between cities, we can now find a sequence that can make the shortest path to all cities A, B, C, D, and E. For example, after calculation, it may be B-->A-->C-->E-->D. In other words, find this order.
Enumeration
If we want to solve this problem first, there are actually many solutions. To put it bluntly, we just need to find an order that can minimize the sum of paths, so the easiest thing to think of is Naturally, it is an enumeration. For example, let A go first and then see if the nearest one to A is B, then go to B, and then go from B. Of course, this is a local greedy strategy, and it is easy to reach the local optimum. Then we can consider DP at this time, that is to say, we still assume that we start from A, and then ensure that 2 cities are the shortest, 3 cities are the shortest, and 4 and 5 are the shortest. Finally, assume the same thing from B. Or directly enumerate all situations and calculate the distance. But no matter what, as the number of cities increases, their complexity will increase, so at this time we have to find ways to make computing use our human expertise. I call it "blindness".
Intelligent Algorithm
Now let’s talk about this intelligent algorithm and why we should use it. We just said that the previous solution will require a large amount of calculations for a large amount of data, which is not necessarily the case. Simple to write. So at this time, first of all, for the TSP problem alone, what we want is a sequence, a sequence that will not repeat. So at this time, is there a simpler solution? And when the data is large enough, we don't necessarily need a completely accurate and completely minimal solution, as long as it's close. So at this time, if we use traditional algorithms, one is one. It will only calculate according to our rules, and we really don’t know what the standard answer is. It is also difficult to set a threshold to stop the calculation for traditional algorithms. . But for us people, there is something called "luck". Some people are so lucky that they may be confused about the answer as soon as they enter their soul. So our intelligent algorithm is actually a bit similar to "Monkey". But people pay attention to skills. For example, experience tells us that three long and one short is the shortest. You can use this technique to guess the answer. Or when you are looking for a boy friend who is as handsome as a blogger, you only need a piece of 40 series. (30 is also okay) The graphics card can be easily taken away. Meng requires skill, we call this strategy.
Strategy
So the technique we just talked about, this trick. In intelligent algorithms, this mask is one of our strategies. How can we get rid of it so that our solution can be more reasonable? Then at this time, a hundred flowers begin to bloom. I won’t chant sutras here. Let’s take the two most classic algorithms as examples, one is the genetic algorithm and the other is the particle swarm algorithm (PSO). As an example, they used a strategy to mask, such as genetic algorithm. By simulating natural selection, they first randomly generated a bunch of solutions and a bunch of sequences, and then based on our natural selection strategy. Screen these solutions, and then use these solutions to find new and better solutions. After going back and forth like this, I finally got a good solution. The particle swarm is similar. We will explain these parts in detail when we use them.
Algorithm
Now that we already know this strategy, what is the algorithm? In fact, it is the steps to implement these strategies, which is our code, our loop, and data structure. We need to realize what we just mentioned, such as natural selection, such as our TSP, how to randomly generate a bunch of solutions.
Data Sample
ok, we have finished talking about some basic concepts here, so at this time, let’s take a look at how we represent this TSP problem. This is actually very simple. , for us here we simply prepare a test data. We assume there are 14 cities here, then our data for these cities are as follows:
data = np.array([16.47, 96.10, 16.47, 94.44, 20.09, 92.54,
22.39, 93.37, 25.23, 97.24, 22.00, 96.05, 20.47, 97.02,
17.20, 96.29, 16.30, 97.38, 14.05, 98.12, 16.53, 97.38,
21.52, 95.59, 19.41, 97.13, 20.09, 92.55]).reshape((14, 2))We will use this set of data for testing later, and now above There are already 14 cities.
Then let’s start our solution
Genetic Algorithm
ok, then let’s talk about what our genetic algorithm is all about, and then , we use this to solve this TSP problem.
So now, let’s take a look at how our genetic algorithm is fooled.
算法流程
遗传算法其实是在用计算机模拟我们的物种进化。其实更加通俗的说法是筛选，这个就和我们袁老爷爷种植水稻一样。有些个体发育良好，有些个体发育不好，那么我就先筛选出发育好的，然后让他们去繁衍后代，然后再筛选，最后得到高产水稻。其实也和我们社会一样，不努力就木有女朋友就不能保留自己的基因，然后剩下的人就是那些优秀的人和富二代的基因，这就是现实呀。所以得好好学习，天天向上！
那么回到主题，我们的遗传算法就是在模拟这一个过程，模拟一个物竞天择的过程。
所以在我们的算法里面也是分为几大块
繁殖
首先我们的种群需要先繁殖。这样才能不断产生优良基于，那么对应我们的算法，假设我们需要求取
Y = np.sin(10 * x) * x + np.cos(2 * x) * x
的最大值（在一个范围内）那么我们的个体就是一组（X1）的解。好的个体就会被保留，不好的就会被pass，选择标准就是我们的函数 Y 。那么问题来了如何模拟这个过程？我们都知道在繁殖后代的时候我们是通过DNA来保留我们的基因信息，在这个过程当中，父母的DNA交互，并且在这个过程当中会产生变异，这样一来，父母双方的优秀基于会被保存，并且产生的变异有可能诞生更加优秀的后代。
所以接下来我们需要模拟我们的ＤＮＡ，进行交叉和变异。
交叉
这个交叉过程和我们的生物其实很像，当然我们在我们的计算机里面对于数字我们可以将其转化为二进制，当做我们的DNA
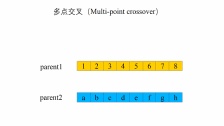
交叉的方式有很多，我们这边选择这一个，进行交叉。
变异
那这个在我们这里就更加简单了
我们只需要在交叉之后，再随机选择几个位置进行改变值就可以了。当然变异的概率是很小的，并且是随机的，这一点要注意。并且由于变异是随机的，所以不排除生成比原来还更加糟糕的个体。
选择
最后我们按照一定的规则去筛选这个些个体就可以了，然后淘汰原来的个体。那么在我们的计算机里面是使用了两个东西，首先我们要把原来二进制的玩意，给转化为我们原来的十进制然后带入我们的函数运算，然后保存起来，之后再每一轮统一筛选一下就好了。
逆转
这个咋说呢，说好听点叫逆转，难听点就算，对于一些新的生成的不好的解，我们是要舍弃的。
代码
那么这部分用代码描述的话就是这样的：
import numpy as np
import matplotlib.pyplot as plt
Population_Size = 100
Iteration_Number = 200
Cross_Rate = 0.8
Mutation_Rate = 0.003
Dna_Size = 10
X_Range=[0,5]
def F(x):
'''
目标函数，需要被优化的函数
:param x:
:return:
'''
return np.sin(10 * x) * x + np.cos(2 * x) * x
def CrossOver(Parent,PopSpace):
'''
交叉DNA，我们直接在种群里面选择一个交配
然后就生出孩子了
:param parent:
:param PopSpace:
:return:
'''
if(np.random.rand()) < Cross_Rate:
cross_place = np.random.randint(0, 2, size=Dna_Size).astype(np.bool)
cross_one = np.random.randint(0, Population_Size, size=1) #选择一位男/女士交配
Parent[cross_place] = PopSpace[cross_one,cross_place]
return Parent
def Mutate(Child):
'''
变异
:param Child:
:return:
'''
for point in range(Dna_Size):
if np.random.rand() < Mutation_Rate:
Child[point] = 1 if Child[point] == 0 else 0
return Child
def TranslateDNA(PopSpace):
'''
把二进制转化为十进制方便计算
:param PopSpace:
:return:
'''
return PopSpace.dot(2 ** np.arange(Dna_Size)[::-1]) / float(2 ** Dna_Size - 1) * X_Range[1]
def Fitness(pred):
'''
这个其实是对我们得到的F(x)进行换算，其实就是选择的时候
的概率，我们需要处理负数，因为概率不能为负数呀
pred 这是一个二维矩阵
:param pred:
:return:
'''
return pred + 1e-3 - np.min(pred)
def Select(PopSpace,Fitness):
'''
选择
:param PopSpace:
:param Fitness:
:return:
'''
'''
这里注意的是，我们先按照权重去选择我们的优良个体，所以我们这里选择的时候允许重复的元素出现
之后我们就可以去掉这些重复的元素，这样才能实现保留良种去除劣种。100--》70（假设有30个重复）
如果不允许重复的话，那你相当于没有筛选
'''
Better_Ones = np.random.choice(np.arange(Population_Size), size=Population_Size, replace=True,
p=Fitness / Fitness.sum())
# np.unique(Better_Ones) #这个是我后面加的
return PopSpace[Better_Ones]
if __name__ == '__main__':
PopSpace = np.random.randint(2, size=(Population_Size, Dna_Size)) # initialize the PopSpace DNA
plt.ion()
x = np.linspace(X_Range, 200)
# plt.plot(x, F(x))
plt.xticks([0,10])
plt.yticks([0,10])
for _ in range(Iteration_Number):
F_values = F(TranslateDNA(PopSpace))
# something about plotting
if 'sca' in globals():
sca.remove()
sca = plt.scatter(TranslateDNA(PopSpace), F_values, s=200, lw=0, c='red', alpha=0.5)
plt.pause(0.05)
# GA part (evolution)
fitness = Fitness(F_values)
print("Most fitted DNA: ", PopSpace[np.argmax(fitness)])
PopSpace = Select(PopSpace, fitness)
PopSpace_copy = PopSpace.copy()
for parent in PopSpace:
child = CrossOver(parent, PopSpace_copy)
child = Mutate(child)
parent[:] = child
plt.ioff()
plt.show()这个代码是以前写的，逆转没有写上（下面的有）
TSP遗传算法
ok,刚刚的例子是拿的解方程，也就是说是一个连续问题吧，当然那个连续处理的话并不是很好，只是一个演示。那么我们这个的话其实类似的。首先我们的ＤＮＡ，是城市的路径，也就是A-B-C-D等等，当然我们用下标表示城市。
种群表示
首先我们确定了使用城市的序号作为我们的个体DNA，例如咱们种群大小为100，有ＡＢＣＤ四个城市，那么他就是这样的，我们先随机生成种群，长这个样：
1 2 3 4
2 3 4 5
3 2 1 4
...
那个1,2,3,4是ABCD的序号。
交叉与变异
这里面的话，值得一提的就是，由于暂定城市需要是不能重复的，且必须是完整的，所以如果像刚刚那样进行交叉或者变异的话，那么实际上会出点问题，我们不允许出现重复，且必须完整，对于我们的DNA，也就是咱们瞎蒙的个体。
代码
由于咱们每一步在代码里面都有注释，所以的话咱们在这里就不再进行复述了。
from math import floor
import numpy as np
import matplotlib.pyplot as plt
class Gena_TSP(object):
"""
使用遗传算法解决TSP问题
"""
def __init__(self, data, maxgen=200,
size_pop=200, cross_prob=0.9,
pmuta_prob=0.01, select_prob=0.8
):
self.maxgen = maxgen # 最大迭代次数
self.size_pop = size_pop # 群体个数，（一次性瞎蒙多少个解）
self.cross_prob = cross_prob # 交叉概率
self.pmuta_prob = pmuta_prob # 变异概率
self.select_prob = select_prob # 选择概率
self.data = data # 城市的坐标数据
self.num = len(data) # 有多少个城市，对应多少个坐标，对应染色体的长度（我们的解叫做染色体）
"""
计算城市的距离，我们用矩阵表示城市间的距离
"""
self.__matrix_distance = self.__matrix_dis()
self.select_num = int(self.size_pop * self.select_prob)
# 通过选择概率确定子代的选择个数
"""
初始化子代和父代种群，两者相互交替
"""
self.parent = np.array([0] * self.size_pop * self.num).reshape(self.size_pop, self.num)
self.child = np.array([0] * self.select_num * self.num).reshape(self.select_num, self.num)
"""
负责计算每一个个体的（瞎蒙的解）最后需要多少距离
"""
self.fitness = np.zeros(self.size_pop)
self.best_fit = []
self.best_path = []
# 保存每一步的群体的最优路径和距离
def __matrix_dis(self):
"""
计算14个城市的距离，将这些距离用矩阵存起来
:return:
"""
res = np.zeros((self.num, self.num))
for i in range(self.num):
for j in range(i + 1, self.num):
res[i, j] = np.linalg.norm(self.data[i, :] - self.data[j, :])
res[j, i] = res[i, j]
return res
def rand_parent(self):
"""
初始化种群
:return:
"""
rand_ch = np.array(range(self.num))
for i in range(self.size_pop):
np.random.shuffle(rand_ch)
self.parent[i, :] = rand_ch
self.fitness[i] = self.comp_fit(rand_ch)
def comp_fit(self, one_path):
"""
计算，咱们这个路径的长度，例如A-B-C-D
:param one_path:
:return:
"""
res = 0
for i in range(self.num - 1):
res += self.__matrix_distance[one_path[i], one_path[i + 1]]
res += self.__matrix_distance[one_path[-1], one_path[0]]
return res
def out_path(self, one_path):
"""
输出我们的路径顺序
:param one_path:
:return:
"""
res = str(one_path[0] + 1) + '-->'
for i in range(1, self.num):
res += str(one_path[i] + 1) + '-->'
res += str(one_path[0] + 1) + '\n'
print(res)
def Select(self):
"""
通过我们的这个计算的距离来计算出概率，也就是当前这些个体DNA也就瞎蒙的解
之后我们在通过概率去选择个体，放到child里面
:return:
"""
fit = 1. / (self.fitness) # 适应度函数
cumsum_fit = np.cumsum(fit)
pick = cumsum_fit[-1] / self.select_num * (np.random.rand() + np.array(range(self.select_num)))
i, j = 0, 0
index = []
while i < self.size_pop and j < self.select_num:
if cumsum_fit[i] >= pick[j]:
index.append(i)
j += 1
else:
i += 1
self.child = self.parent[index, :]
def Cross(self):
"""
模仿DNA交叉嘛，就是交换两个瞎蒙的解的部分的解例如
A-B-C-D
C-D-A-B
我们选几个交叉例如这样
A-D-C-B
1,3号交换了位置，当然这里注意可不能重复啊
:return:
"""
if self.select_num % 2 == 0:
num = range(0, self.select_num, 2)
else:
num = range(0, self.select_num - 1, 2)
for i in num:
if self.cross_prob >= np.random.rand():
self.child[i, :], self.child[i + 1, :] = self.intercross(self.child[i, :],
self.child[i + 1, :])
def intercross(self, ind_a, ind_b):
"""
这个是我们两两交叉的具体实现
:param ind_a:
:param ind_b:
:return:
"""
r1 = np.random.randint(self.num)
r2 = np.random.randint(self.num)
while r2 == r1:
r2 = np.random.randint(self.num)
left, right = min(r1, r2), max(r1, r2)
ind_a1 = ind_a.copy()
ind_b1 = ind_b.copy()
for i in range(left, right + 1):
ind_a2 = ind_a.copy()
ind_b2 = ind_b.copy()
ind_a[i] = ind_b1[i]
ind_b[i] = ind_a1[i]
x = np.argwhere(ind_a == ind_a[i])
y = np.argwhere(ind_b == ind_b[i])
if len(x) == 2:
ind_a[x[x != i]] = ind_a2[i]
if len(y) == 2:
ind_b[y[y != i]] = ind_b2[i]
return ind_a, ind_b
def Mutation(self):
"""
之后是变异模块，这个就是按照某个概率，去替换瞎蒙的解里面的其中几个元素。
:return:
"""
for i in range(self.select_num):
if np.random.rand() <= self.cross_prob:
r1 = np.random.randint(self.num)
r2 = np.random.randint(self.num)
while r2 == r1:
r2 = np.random.randint(self.num)
self.child[i, [r1, r2]] = self.child[i, [r2, r1]]
def Reverse(self):
"""
近化逆转，就是说下一次瞎蒙的解如果没有更好的话就不进入下一代，同时也是随机选择一个部分的
我们不是一次性全部替换
:return:
"""
for i in range(self.select_num):
r1 = np.random.randint(self.num)
r2 = np.random.randint(self.num)
while r2 == r1:
r2 = np.random.randint(self.num)
left, right = min(r1, r2), max(r1, r2)
sel = self.child[i, :].copy()
sel[left:right + 1] = self.child[i, left:right + 1][::-1]
if self.comp_fit(sel) < self.comp_fit(self.child[i, :]):
self.child[i, :] = sel
def Born(self):
"""
替换，子代变成新的父代
:return:
"""
index = np.argsort(self.fitness)[::-1]
self.parent[index[:self.select_num], :] = self.child
def main(data):
Path_short = Gena_TSP(data) # 根据位置坐标，生成一个遗传算法类
Path_short.rand_parent() # 初始化父类
## 绘制初始化的路径图
fig, ax = plt.subplots()
x = data[:, 0]
y = data[:, 1]
ax.scatter(x, y, linewidths=0.1)
for i, txt in enumerate(range(1, len(data) + 1)):
ax.annotate(txt, (x[i], y[i]))
res0 = Path_short.parent[0]
x0 = x[res0]
y0 = y[res0]
for i in range(len(data) - 1):
plt.quiver(x0[i], y0[i], x0[i + 1] - x0[i], y0[i + 1] - y0[i], color='r', width=0.005, angles='xy', scale=1,
scale_units='xy')
plt.quiver(x0[-1], y0[-1], x0[0] - x0[-1], y0[0] - y0[-1], color='r', width=0.005, angles='xy', scale=1,
scale_units='xy')
plt.show()
print('初始染色体的路程: ' + str(Path_short.fitness[0]))
# 循环迭代遗传过程
for i in range(Path_short.maxgen):
Path_short.Select() # 选择子代
Path_short.Cross() # 交叉
Path_short.Mutation() # 变异
Path_short.Reverse() # 进化逆转
Path_short.Born() # 子代插入
# 重新计算新群体的距离值
for j in range(Path_short.size_pop):
Path_short.fitness[j] = Path_short.comp_fit(Path_short.parent[j, :])
index = Path_short.fitness.argmin()
if (i + 1) % 50 == 0:
print('第' + str(i + 1) + '步后的最短的路程: ' + str(Path_short.fitness[index]))
print('第' + str(i + 1) + '步后的最优路径:')
Path_short.out_path(Path_short.parent[index, :]) # 显示每一步的最优路径
# 存储每一步的最优路径及距离
Path_short.best_fit.append(Path_short.fitness[index])
Path_short.best_path.append(Path_short.parent[index, :])
return Path_short # 返回遗传算法结果类
if __name__ == '__main__':
data = np.array([16.47, 96.10, 16.47, 94.44, 20.09, 92.54,
22.39, 93.37, 25.23, 97.24, 22.00, 96.05, 20.47, 97.02,
17.20, 96.29, 16.30, 97.38, 14.05, 98.12, 16.53, 97.38,
21.52, 95.59, 19.41, 97.13, 20.09, 92.55]).reshape((14, 2))
main(data)运行结果
ok,我们来看看运行的结果：
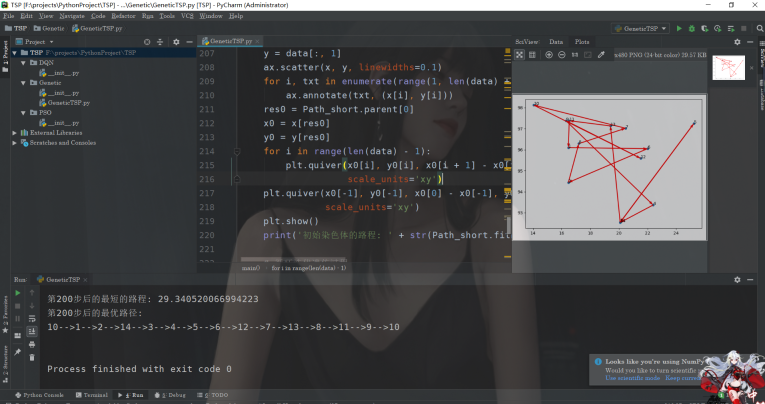
The above is the detailed content of How to use Python to implement genetic algorithm to solve the Traveling Salesman Problem (TSP)?. For more information, please follow other related articles on the PHP Chinese website!