Detailed explanation of binary tree traversal and methods of logical operations

First of all, we still have to explain that the main content of our study is binary trees, because binary trees are the most typical application of trees. Whether it is an exam or an interview, it is a must-know and must-learn content.

First of all, before learning the operation of the tree, we must first understand that the core of the operation of the tree is "traversal". Why do you say that? Unlike stacks and queues, the tree structure is actually no longer one-dimensional. It has branches, different angles, and more importantly, it has the concept of hierarchy.

The thing in one-dimensional space is our common "line", which has only length but no height, and this length is its only dimension. Stacks and queues are obviously one-dimensional. The tree is different. Because of the concept of hierarchy, it has a "height", that is, it has been upgraded to a two-dimensional concept. Just like the bunch of nouns introduced in the previous article, there is the concept of "height (depth) of a tree".

After being able to traverse a tree, we can add, delete, modify and other operations on the nodes of the tree based on the traversal. These basic logical operations are all based on the traversal. Above, think carefully about stacks and queues. In fact, don’t their logical operations also start with traversal? Whether it is popping or entering the stack or dequeuing or enqueuing, we are all based on a fixed traversal rule (FILO, FIFO).

For two-dimensional things, how to traverse it is a key point. We only need to traverse the one-dimensional data structure sequentially, but the two-dimensional data results cannot simply be traversed one by one in order, because there are hierarchical relationships between nodes, so we have to consider the current If the node has no child nodes, what should we do during the traversal operation?

Fortunately, we learn this knowledge by standing on the shoulders of giants. Many seniors have summarized some very simple tree traversal methods for us. How simple are they? Let’s get out of the way first, let’s take a look at how to build a tree, which is the binary tree we showed in the previous article.

Chained storage structure of binary trees

Using chained storage of binary trees is very simple and very visual. Friends, let’s put away the sequential storage of binary trees. question, because in the next article we will explain under what circumstances sequential storage is used.

class BiTree
{
    public $data;
    public $lChild;
    public $rChild;
}

In fact, in chain storage, we use nodes one by one to save the tree. Each binary tree node has a data field, which is the data attribute. The other two attributes can be regarded as two forked pointers, namely the left child node lChild and the right child node rChild of this node.

Comparing the stack and the queue, we just replaced the next node with the left and right child nodes. In essence, it is not much different from the stack and the queue. To put it bluntly, from the perspective of data structure, we still use one-dimensional storage to represent two-dimensional concepts, and the transformation of this concept requires us to start from the perspective of conceptual understanding.

Binary Tree Building

// 建立二叉树
function CreateBiTree($arr, $i)
{
    if (!isset($arr[$i])) {
        return null;
    }
    $t = new BiTree();
    $t->data = $arr[$i];
    $t->lChild = CreateBiTree($arr, $i * 2);
    $t->rChild = CreateBiTree($arr, $i * 2 + 1);
    return $t;
}

With such a simple method, we can complete the establishment of a chained binary tree. Friends, please watch carefully. This simple tree-building operation actually contains a lot of mysteries:

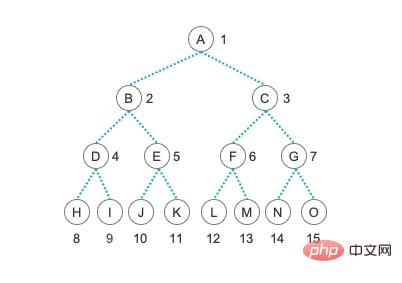
• We use an array to represent each node of the tree in sequence, for example, enter A in sequence , B, C, D, E... (We will see them again in the sequential storage of the tree)

• The content of the assignment is the data of the current $i subscript, please note that we A recursive operation was performed when assigning values ​​to the left and right children

• When learning the stack, we learned that "recursion" is a stack-type operation, so in this code , we build the tree in the form of a stack

• Did you notice i 2 and i 2 1 each time? Please review the properties of binary trees 5

Finally, we test whether this method can successfully build a linked tree structure.

$treeList = ['', 'A', 'B', 'C', 'D', 'E', 'F', 'G', 'H', 'I', 'J', 'K', 'L', 'M', 'N', 'O'];

$tree = CreateBiTree($treeList, 1);
print_r($tree);

// BiTree Object
// (
//     [data] => A
//     [lChild] => BiTree Object
//         (
//             [data] => B
//             [lChild] => BiTree Object
//                 (
//                     [data] => D
//                     [lChild] => BiTree Object
//                         (
//                             [data] => H
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                     [rChild] => BiTree Object
//                         (
//                             [data] => I
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                 )

//             [rChild] => BiTree Object
//                 (
//                     [data] => E
//                     [lChild] => BiTree Object
//                         (
//                             [data] => J
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                     [rChild] => BiTree Object
//                         (
//                             [data] => K
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                 )

//         )

//     [rChild] => BiTree Object
//         (
//             [data] => C
//             [lChild] => BiTree Object
//                 (
//                     [data] => F
//                     [lChild] => BiTree Object
//                         (
//                             [data] => L
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                     [rChild] => BiTree Object
//                         (
//                             [data] => M
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                 )

//             [rChild] => BiTree Object
//                 (
//                     [data] => G
//                     [lChild] => BiTree Object
//                         (
//                             [data] => N
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                     [rChild] => BiTree Object
//                         (
//                             [data] => O
//                             [lChild] =>
//                             [rChild] =>
//                         )

//                 )

//         )

// )

The printed content should be very clear, right? Node A has two left and right child nodes, namely B and C. Node B has two left and right children, namely D and E, and so on. The final structure is exactly the same as the structure of our binary tree diagram above. Here, we also need to pay attention to the fact that for the array passed in, we give the first element, that is, the data with a 0 subscript, empty, and build the tree starting from the second element, which is the element with a 1 subscript. This is also to be able to intuitively and conveniently use Property 5 of binary trees to quickly build this tree.

二叉树的遍历

说完二叉树的建树了，其实我们就已经接触到了一种二叉树的遍历形式。注意看我们建树方法中的代码，我们是先给结点的 data 赋值，然后建立这个结点的左、右孩子结点，并为它们赋值后再继续使用同样的操作一路建立完成所有的结点。现在，我们将这个操作反过来，不是建立结点，而是读取这些结点的内容，先读取结点的内容，然后再读取这个结点左右孩子结点的内容，这就是“先序遍历”。

先序遍历

/**
 * 前序遍历
 */
function PreOrderTraverse(?BiTree $t)
{
    if ($t) {
        echo $t->data, ',';
        PreOrderTraverse($t->lChild);
        PreOrderTraverse($t->rChild);
    }
}

PreOrderTraverse($tree);

// A,B,D,H,I,E,J,K,C,F,L,M,G,N,O,

是不是很神奇？就连建树我们竟然也使用的是同一种遍历的方法，可以看出对于二叉树这种复杂的数据结构来说，遍历的重要作用了吧。

大家可以看一个遍历读取出来的结点顺序，貌似和我们输入的顺序不一样呀！没错，先序遍历是通过递归，先按一个方向走到底，当这个结点没有子结点之后，通过递归栈的特性再向上弹出。并且在遍历孩子结点之前先输出当前这个结点的内容。注意，这一句话很重要！所以我们的顺序就是 A,B,D,H ，当 H 没有子结点之后，我们就回到父结点 D 再进入它的右子结点 I ，具体顺序可以参考下图：

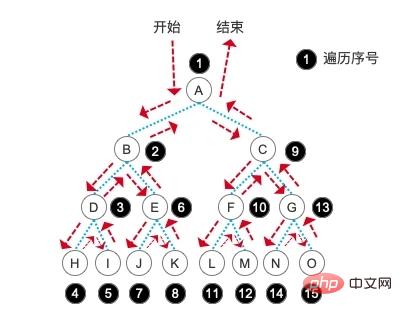
我们代码中的先序遍历和先序建树的结点顺序是完全不一样的，这一点也是要搞清楚的。建树的过程我们根据二叉树的 性质5 直接为它指定了数据下标。而在遍历过程中则是一个结点一个结点的去扫描遍历整颗树的。

中序遍历

顾名思义，中序遍历其实就是在遍历完左孩子结点之后再输出当前这个结点的内容，所以我们只需要微调先序遍历的代码即可。

/**
 * 中序遍历
 */
function InOrderTraverse(?BiTree $t)
{
    if ($t) {
        InOrderTraverse($t->lChild);
        echo $t->data, ',';
        InOrderTraverse($t->rChild);
    }
}

InOrderTraverse($tree);

// H,D,I,B,J,E,K,A,L,F,M,C,N,G,O,

中序遍历的步骤就是我们会直接先走到最左边的子结点，当遇到最后一个结点时，输出内容，也就是图中的 H 结点，接着回到它的父结点 D 结点，这时根据中序的原理输出 D ，再进入它的右孩子结点并输出 I 。

D 结点的子树及它本身遍历完成后，返回 D 结点的上级结点 B 结点，输出 B ，然后进入 B 结点的右孩子结点 E 。再次进入到 E 的最左孩子结点 J ，然后参考 D 结点的遍历形式完成整颗树的遍历。具体顺序参考下图：

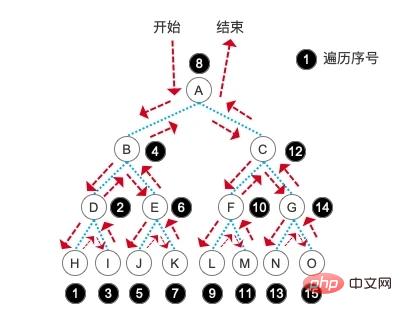
后序遍历

在学习了先序和中序之后，从名字就可以看出来后序就是在遍历完一个结点的左右孩子之后最后输出这个结点的内容，代码当然也是简单地微调一下就可以了。

/**
 * 后序遍历
 */
function PostOrderTraverse(?BiTree $t)
{
    if ($t) {
        PostOrderTraverse($t->lChild);
        PostOrderTraverse($t->rChild);
        echo $t->data, ',';
    }
}

PostOrderTraverse($tree);

// H,I,D,J,K,E,B,L,M,F,N,O,G,C,A,

具体原理就不详细说明了，相信在学习了先序和中序之后，你一定能马上想明白后序遍历到底是什么意思了。直接上图：

层序遍历

最后，我们要讲的就是层序遍历。既然有“层”这个关键字了，相信大家马上就能联想到，是不是一层一层地去遍历啊！没错，层序遍历就是这个意思，我们按照树的层次，一层一层地输出相应的结点信息。需要注意的，在这里我们会用到队列，而不是栈了。

/**
 * 层序遍历
 */
$q = InitLinkQueue();
function LevelOrderTraverse(?BiTree $t)
{
    global $q;
    if (!$t) {
        return;
    }

    EnLinkQueue($q, $t);
    $node = $q;
    while ($node) {
        $node = DeLinkQueue($q);
        if ($node->lChild) {
            EnLinkQueue($q, $node->lChild);
        }
        if ($node->rChild) {
            EnLinkQueue($q, $node->rChild);
        }
        echo $node->data, ',';
    }
}

LevelOrderTraverse($tree);

// A,B,C,D,E,F,G,H,I,J,K,L,M,N,O,

InitLinkQueue() EnLinkQueue() 、 EnLinkQueue() 这些都是我们之前学习队列的时候所写的对于队列的逻辑操作方法。是不是很开心呀，之前的知识又用上了。层序遍历的核心思想就是运用队列的概念，遇到一个结点，就把这个结点入队，然后判断它是否有子结点，然后相继把子结点入队。

每遍历一个结点，就把队首的结点出队，这样就完成了按树的层次遍历的能力。文字说明还是太抽象，我们还是通过图片来展示这一过程：

大家有没有发现，层序遍历的输出结果就和我们建树时的数组顺序完全相同了。很好玩吧，所以说代码的世界总是有无穷的乐趣等着我们去发现哦！

总结

今天的内容有没有懵圈？如果懵圈了就多找资料好好研究一下，先序、中序、后序都是利用栈来进行树的结点遍历的，而层序遍历则是利用了队列。一环套一环呀，前面学习的内容都派上用场了吧！不过这只是个开始，在学习图的时候，我们会在深度遍历和广度遍历中再次看到栈和队列的身影，它们可都是亲戚哦。

这四种遍历方式在考试和面试中也是经常出现的，不管是它们的原理还是画图或者是根据图形来写出各种遍历的顺序，都是非常常见的考核内容，所以大家在这篇文章入门的基础上还是要更加深入的去根据一些教材来深入的理解这几种遍历，熟练的掌握它们。

测试代码：

https://github.com/zhangyue0503/Data-structure-and-algorithm/blob/master/4.树/source/4.2二叉树的遍历及逻辑操作.php

推荐学习：php视频教程

The above is the detailed content of Detailed explanation of binary tree traversal and methods of logical operations. For more information, please follow other related articles on the PHP Chinese website!