Home >Web Front-end >JS Tutorial >Binary tree, dynamic programming and backtracking method in JavaScript (case analysis)
Binary tree, dynamic programming and backtracking method in JavaScript (case analysis)
- angryTomforward
- 2019-11-28 13:21:242453browse
I wrote it in a hurry. All the test cases can be run through. However, considering memory and efficiency, there are still many areas that need improvement, so please give me more advice

Problem description
Given a binary tree, the root node is level 1 and the depth is 1. Append a row of nodes with value v to its dth level.
Add rules: Given a depth value d (positive integer), for each non-empty node N of the depth d-1 layer, create two left subtrees and right subtrees with values v for N Tree.
Connect the original left subtree of N to the left subtree of the new node v;
Connect the original right subtree of N to the right subtree of the new node v.
If the value of d is 1 and the depth d - 1 does not exist, a new root node v will be created, and the original entire tree will serve as the left subtree of v.
Example
[Related course recommendations: JavaScript video tutorial]
Input: A binary tree as following: 4 / \ 2 6 / \ / 3 1 5 v = 1d = 2Output: 4 / \ 1 1 / \ 2 6 / \ / 3 1 5
Basic idea
Pre-order traversal of a binary tree
The basic structure of the code
is not the final structure, but the general structure
/**
* @param {number} cd:current depth,递归当前深度
* @param {number} td:target depth, 目标深度
*/
var traversal = function (node, v, cd, td) {
// 递归到目标深度,创建新节点并返回
if (cd === td) {
// return 新节点
}
// 向左子树递归
if (node.left) {
node.left = traversal (node.left, v, cd + 1, td);
}
// 向右子树递归
if (node.right) {
node.right = traversal (node.right, v, cd + 1, td);
}
// 返回旧节点
return node;
};
/**
* Definition for a binary tree node.
* function TreeNode(val) {
* this.val = val;
* this.left = this.right = null;
* }
*/
/**
* @param {TreeNode} root
* @param {number} v
* @param {number} d
* @return {TreeNode}
*/
var addOneRow = function (root, v, td) {
// 从根节点开始递归
traversal (root, v, 1, td);
return root;
};
Specific analysis
We can classify the discussion and handle it in three situations
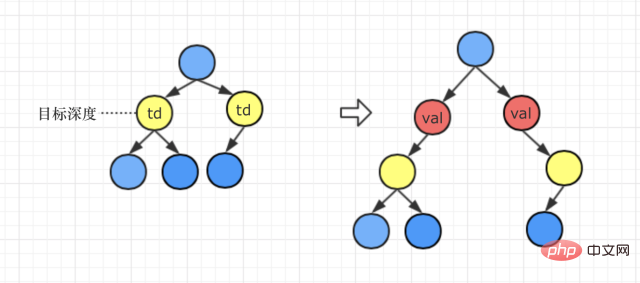
The first situation: target depth
Processing method: The val node replaces the node corresponding to the target depth, and
●If the target node is originally the left subtree, then the target node is the left of the val node after reset Subtree
● If the target node was originally the right subtree, then after reset the target node is the right subtree of the val node
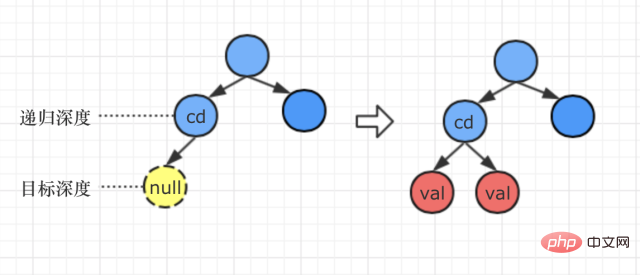
2 situations: target depth > the maximum depth of the current recursive path
After reading the question, I found that there is such a description: "The range of the input depth value d is: [1, the maximum depth of the binary tree is 1] ”
So, when the target depth happens to be one layer deeper than the depth of the tree of the current path, the processing method is:
Add val to the left and right branches of the bottom layer node Node
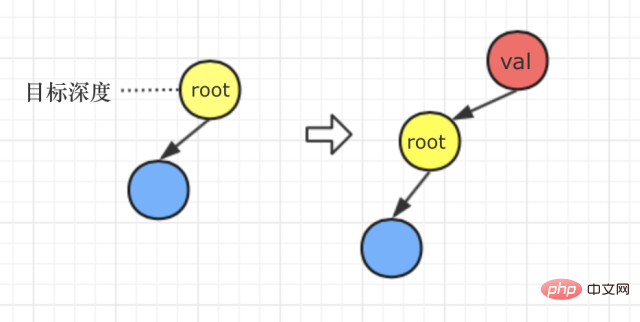
Case 3: The target depth is 1
Let’s analyze the meaning of the question again. The question says: “If d The value of is 1 and the depth d - 1 does not exist, then create a new root node v, and the original entire tree will be used as the left subtree of v."
This shows that when: the target depth is 1 , our processing method is like this
/**
* @param {v} val,插入节点携带的值
* @param {cd} current depth,递归当前深度
* @param {td} target depth, 目标深度
* @param {isLeft} 判断原目标深度的节点是在左子树还是右子树
*/
var traversal = function (node, v, cd, td, isLeft) {
debugger;
if (cd === td) {
const newNode = new TreeNode (v);
// 如果原来是左子树,重置后目标节点还是在左子树上,否则相反
if (isLeft) {
newNode.left = node;
} else {
newNode.right = node;
}
return newNode;
}
// 处理上述的第1和第2种情况
if (node.left || (node.left === null && cd + 1 === td)) {
node.left = traversal (node.left, v, cd + 1, td, true);
}
if (node.right || (node.right === null && cd + 1 === td)) {
node.right = traversal (node.right, v, cd + 1, td, false);
}
return node;
};
/**
* Definition for a binary tree node.
* function TreeNode(val) {
* this.val = val;
* this.left = this.right = null;
* }
*/
/**
* @param {TreeNode} root
* @param {number} v
* @param {number} d
* @return {TreeNode}
*/
var addOneRow = function (root, v, td) {
// 处理目标深度为1的情况,也就是上述的第3种情况
if (td === 1) {
const n = new TreeNode (v);
n.left = root;
return n;
}
traversal (root, v, 1, td);
return root;
};
Word splitting
Given a non-empty string s and a dictionary wordDict containing a non-empty word list, determine whether s can be split by spaces into one or more words that appear in the dictionary. Instructions: 1. Words in the dictionary can be reused when splitting.2.你可以假设字典中没有重复的单词。
Example
example1 输入: s = "applepenapple", wordDict = ["apple", "pen"] 输出: true解释: 返回 true 因为 "applepenapple" 可以被拆分成 "apple pen apple"。 注意: 你可以重复使用字典中的单词。 example2 输入: s = "catsandog", wordDict = ["cats", "dog", "sand", "and", "cat"] 输出: false来源:力扣(LeetCode) 链接:https://leetcode-cn.com/problems/word-break
基本思想
动态规划
具体分析
动态规划的关键点是:寻找状态转移方程
有了这个状态转移方程,我们就可以根据上一阶段状态和决策结果,去求出本阶段的状态和结果
然后,就可以从初始值,不断递推求出最终结果。
在这个问题里,我们使用一个一维数组来存放动态规划过程的递推数据
假设这个数组为dp,数组元素都为true或者false,
dp[N] 存放的是字符串s中从0到N截取的子串是否是“可拆分”的布尔值
让我们从一个具体的中间场景出发来思考计算过程
假设我们有
wordDict = ['ab','cd','ef'] s ='abcdef'
并且假设目前我们已经得出了N=1到N=5的情况,而现在需要计算N=6的情况
或者说,我们已经求出了dp[1] 到dp[5]的布尔值,现在需要计算dp[6] = ?
该怎么计算呢?
现在新的字符f被加入到序列“abcde”的后面,如此以来,就新增了以下几种6种需要计算的情况
A序列 + B序列1.abcdef + "" 2.abcde + f3.abcd + ef4.abc + def5.ab + cdef6.a + bcdef 注意:当A可拆且B可拆时,则A+B也是可拆分的
从中我们不难发现两点
1. 当A可拆且B可拆时,则A+B也是可拆分的
2. 这6种情况只要有一种组合序列是可拆分的,abcdef就一定是可拆的,也就得出dp[6] = true了
下面是根据根据已有的dp[1] 到dp[5]的布尔值,动态计算dp[6] 的过程
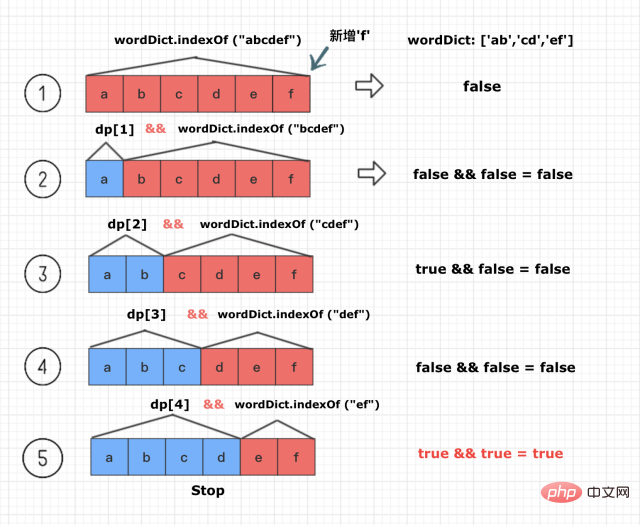
(注意只要计算到可拆,就可以break循环了)
具体代码
var initDp = function (len) {
let dp = new Array (len + 1).fill (false);
return dp;
};
/**
* @param {string} s
* @param {string[]} wordDict
* @return {boolean}
*/
var wordBreak = function (s, wordDict) {
// 处理空字符串
if (s === '' && wordDict.indexOf ('') === -1) {
return false;
}
const len = s.length;
// 默认初始值全部为false
const dp = initDp (len);
const a = s.charAt (0);
// 初始化动态规划的初始值
dp[0] = wordDict.indexOf (a) === -1 ? false : true;
dp[1] = wordDict.indexOf (a) === -1 ? false : true;
// i:end
// j:start
for (let i = 1; i < len; i++) {
for (let j = 0; j <= i; j++) {
// 序列[0,i] = 序列[0,j] + 序列[j,i]
// preCanBreak表示序列[0,j]是否是可拆分的
const preCanBreak = dp[j];
// 截取序列[j,i]
const str = s.slice (j, i + 1);
// curCanBreak表示序列[j,i]是否是可拆分的
const curCanBreak = wordDict.indexOf (str) !== -1;
// 情况1: 序列[0,j]和序列[j,i]都可拆分,那么序列[0,i]肯定也是可拆分的
const flag1 = preCanBreak && curCanBreak;
// 情况2: 序列[0,i]本身就存在于字典中,所以是可拆分的
const flag2 = curCanBreak && j === 0;
if (flag1 || flag2) {
// 设置bool值,本轮计算结束
dp[i + 1] = true;
break;
}
}
}
// 返回最后结果
return dp[len];
};全排列
题目描述
给定一个没有重复数字的序列,返回其所有可能的全排列。
Example
输入: [1,2,3] 输出: [ [1,2,3], [1,3,2], [2,1,3], [2,3,1], [3,1,2], [3,2,1] ]
基本思想
回溯法
具体分析
1. 深度优先搜索搞一波,index在递归中向前推进
2. 当index等于数组长度的时候,结束递归,收集到results中(数组记得要深拷贝哦)
3. 两次数字交换的运用,计算出两种情况
总结
想不通没关系,套路一波就完事了
具体代码
var swap = function (nums, i, j) {
const temp = nums[i];
nums[i] = nums[j];
nums[j] = temp;
};
var recursion = function (nums, results, index) {
// 剪枝
if (index >= nums.length) {
results.push (nums.concat ());
return;
}
// 初始化i为index
for (let i = index; i < nums.length; i++) {
// index 和 i交换??
// 统计交换和没交换的两种情况
swap (nums, index, i);
recursion (nums, results, index + 1);
swap (nums, index, i);
}
};
/**
* @param {number[]} nums
* @return {number[][]}
*/
var permute = function (nums) {
const results = [];
recursion (nums, results, 0);
return results;
};
本文来自 js教程 栏目,欢迎学习!
The above is the detailed content of Binary tree, dynamic programming and backtracking method in JavaScript (case analysis). For more information, please follow other related articles on the PHP Chinese website!

