Linear algebra is fundamental to advanced mathematics and crucial in fields like data science, machine learning, computer vision, and engineering. Eigenvectors, often paired with eigenvalues, are a core concept. This article provides a clear explanation of eigenvectors and their significance.

Table of Contents:
- What are Eigenvectors?
- Understanding Eigenvectors Intuitively
- The Importance of Eigenvectors
- Calculating Eigenvectors
- Eigenvectors in Practice: An Example
- Python Implementation
- Visualizing Eigenvectors
- Summary
- Frequently Asked Questions
What are Eigenvectors?
An eigenvector is a special vector associated with a square matrix. When the matrix transforms the eigenvector, the eigenvector's direction remains unchanged; only its scale is altered by a scalar value called the eigenvalue.
Mathematically, for a square matrix A, a non-zero vector v is an eigenvector if:

Where:
- A is the matrix.
- v is the eigenvector.
- λ (lambda) is the eigenvalue (a scalar).
Understanding Eigenvectors Intuitively
Consider a matrix A representing a linear transformation (e.g., stretching, rotating, or scaling a 2D space). Applying this transformation to a vector v:
- Most vectors will change both direction and magnitude.
- However, some vectors only change in scale (magnitude), not direction. These are eigenvectors.
For instance:
- λ > 1: The eigenvector is stretched.
- 0
- λ = 0: The eigenvector is mapped to the zero vector.
- λ
The Importance of Eigenvectors
Eigenvectors are vital in various applications:
- Principal Component Analysis (PCA): Used for dimensionality reduction, eigenvectors define principal components, capturing maximum variance and identifying key features.
- Google's PageRank: The algorithm uses eigenvectors of a link matrix to determine webpage importance.
- Quantum Mechanics: Eigenvectors and eigenvalues describe system states and measurable properties (e.g., energy levels).
- Computer Vision: Used in facial recognition (e.g., Eigenfaces) to represent images as linear combinations of key features.
- Vibrational Analysis (Engineering): Eigenvectors describe vibration modes in structures (bridges, buildings).
Calculating Eigenvectors
To find eigenvectors:
- Eigenvalue Equation: Start with Av = λv, rewritten as (A - λI)v = 0, where I is the identity matrix.
- Solve for Eigenvalues: Calculate det(A - λI) = 0 to find eigenvalues λ.
- Find Eigenvectors: Substitute each eigenvalue λ into (A - λI)v = 0 and solve for v.
Eigenvectors in Practice: An Example
Given matrix:

- Find Eigenvalues λ: Solve det(A - λI) = 0.
- Find Eigenvectors: Substitute each λ into (A - λI)v = 0 and solve for v.
Python Implementation
Using NumPy:
import numpy as np
A = np.array([[2, 1], [1, 2]])
eigenvalues, eigenvectors = np.linalg.eig(A)
print("Eigenvalues:", eigenvalues)
print("Eigenvectors:", eigenvectors)
Visualizing Eigenvectors
Matplotlib can visualize how eigenvectors transform. (Code omitted for brevity, but the original code provides a good example).
Summary
Eigenvectors are a crucial linear algebra concept with broad applications. They reveal how a matrix transformation affects specific directions, making them essential in various fields. Python libraries simplify eigenvector computation and visualization.
Frequently Asked Questions
- Q1: Eigenvalues vs. Eigenvectors? Eigenvalues are scalars indicating the scaling factor of an eigenvector during a transformation; eigenvectors are the vectors whose direction remains unchanged.
- Q2: Do all matrices have eigenvectors? No, only square matrices can have them, and some square matrices may lack a full set.
- Q3: Are eigenvectors unique? No, any scalar multiple of an eigenvector is also an eigenvector.
- Q4: Eigenvectors in machine learning? Used in PCA for dimensionality reduction.
- Q5: What if an eigenvalue is zero? The corresponding eigenvector is mapped to the zero vector, often indicating a singular matrix.
The above is the detailed content of What is an Eigenvector and Eigenvalue?. For more information, please follow other related articles on the PHP Chinese website!
 How to Run LLM Locally Using LM Studio? - Analytics VidhyaApr 19, 2025 am 11:38 AM
How to Run LLM Locally Using LM Studio? - Analytics VidhyaApr 19, 2025 am 11:38 AMRunning large language models at home with ease: LM Studio User Guide In recent years, advances in software and hardware have made it possible to run large language models (LLMs) on personal computers. LM Studio is an excellent tool to make this process easy and convenient. This article will dive into how to run LLM locally using LM Studio, covering key steps, potential challenges, and the benefits of having LLM locally. Whether you are a tech enthusiast or are curious about the latest AI technologies, this guide will provide valuable insights and practical tips. Let's get started! Overview Understand the basic requirements for running LLM locally. Set up LM Studi on your computer
 Guy Peri Helps Flavor McCormick's Future Through Data TransformationApr 19, 2025 am 11:35 AM
Guy Peri Helps Flavor McCormick's Future Through Data TransformationApr 19, 2025 am 11:35 AMGuy Peri is McCormick’s Chief Information and Digital Officer. Though only seven months into his role, Peri is rapidly advancing a comprehensive transformation of the company’s digital capabilities. His career-long focus on data and analytics informs
 What is the Chain of Emotion in Prompt Engineering? - Analytics VidhyaApr 19, 2025 am 11:33 AM
What is the Chain of Emotion in Prompt Engineering? - Analytics VidhyaApr 19, 2025 am 11:33 AMIntroduction Artificial intelligence (AI) is evolving to understand not just words, but also emotions, responding with a human touch. This sophisticated interaction is crucial in the rapidly advancing field of AI and natural language processing. Th
 12 Best AI Tools for Data Science Workflow - Analytics VidhyaApr 19, 2025 am 11:31 AM
12 Best AI Tools for Data Science Workflow - Analytics VidhyaApr 19, 2025 am 11:31 AMIntroduction In today's data-centric world, leveraging advanced AI technologies is crucial for businesses seeking a competitive edge and enhanced efficiency. A range of powerful tools empowers data scientists, analysts, and developers to build, depl
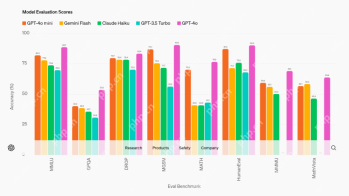 AV Byte: OpenAI's GPT-4o Mini and Other AI InnovationsApr 19, 2025 am 11:30 AM
AV Byte: OpenAI's GPT-4o Mini and Other AI InnovationsApr 19, 2025 am 11:30 AMThis week's AI landscape exploded with groundbreaking releases from industry giants like OpenAI, Mistral AI, NVIDIA, DeepSeek, and Hugging Face. These new models promise increased power, affordability, and accessibility, fueled by advancements in tr
 Perplexity's Android App Is Infested With Security Flaws, Report FindsApr 19, 2025 am 11:24 AM
Perplexity's Android App Is Infested With Security Flaws, Report FindsApr 19, 2025 am 11:24 AMBut the company’s Android app, which offers not only search capabilities but also acts as an AI assistant, is riddled with a host of security issues that could expose its users to data theft, account takeovers and impersonation attacks from malicious
 Everyone's Getting Better At Using AI: Thoughts On Vibe CodingApr 19, 2025 am 11:17 AM
Everyone's Getting Better At Using AI: Thoughts On Vibe CodingApr 19, 2025 am 11:17 AMYou can look at what’s happening in conferences and at trade shows. You can ask engineers what they’re doing, or consult with a CEO. Everywhere you look, things are changing at breakneck speed. Engineers, and Non-Engineers What’s the difference be
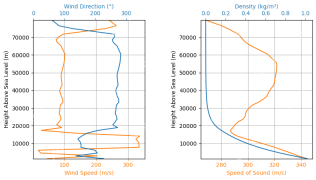 Rocket Launch Simulation and Analysis using RocketPy - Analytics VidhyaApr 19, 2025 am 11:12 AM
Rocket Launch Simulation and Analysis using RocketPy - Analytics VidhyaApr 19, 2025 am 11:12 AMSimulate Rocket Launches with RocketPy: A Comprehensive Guide This article guides you through simulating high-power rocket launches using RocketPy, a powerful Python library. We'll cover everything from defining rocket components to analyzing simula


Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

SublimeText3 Chinese version
Chinese version, very easy to use

VSCode Windows 64-bit Download
A free and powerful IDE editor launched by Microsoft

EditPlus Chinese cracked version
Small size, syntax highlighting, does not support code prompt function

WebStorm Mac version
Useful JavaScript development tools

MinGW - Minimalist GNU for Windows
This project is in the process of being migrated to osdn.net/projects/mingw, you can continue to follow us there. MinGW: A native Windows port of the GNU Compiler Collection (GCC), freely distributable import libraries and header files for building native Windows applications; includes extensions to the MSVC runtime to support C99 functionality. All MinGW software can run on 64-bit Windows platforms.





