本篇文章给大家带来了关于javascript的相关知识,主要介绍了JavaScript二叉树及各种遍历算法详情,文章围绕主题展开详细的内容介绍,具有一定的参考价值,需要的小伙伴可以参考一下,希望对大家有帮助。

【相关推荐:javascript视频教程、web前端】
什么是二叉树
二叉树是每个节点最多只能有两个子节点的树,如下图所示:
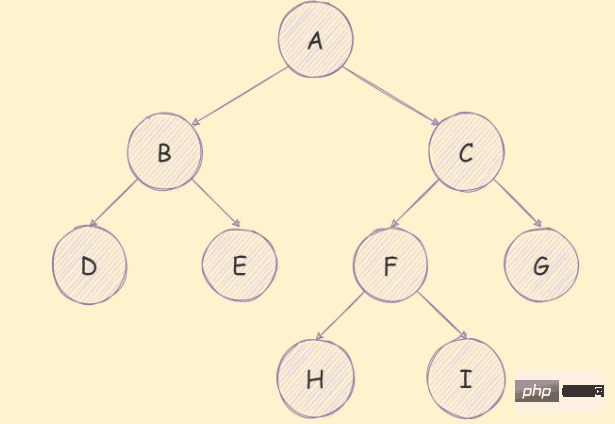
一个二叉树具有以下几个特质:
- 第
i层的节点最有只有2^(i-1)个; - 如果这颗二叉树的深度为
k,那二叉树最多有2^k-1个节点; - 在一个非空的二叉树中,若使用
n0表示叶子节点的个数,n2是度为2的非叶子节点的个数,那么两者满足关系n0 = n2 + 1。
满二叉树
如果在一个二叉树中,除了叶子节点,其余的节点的每个度都是2,则说明该二叉树是一个满二叉树,
如下图所示:
满二叉树除了满足普通二叉树特质,还具有如下几个特质:
- 满二叉树的的第
n层具有2^(n-1)个节点; - 深度为
k的满二叉树一定存在2^k-1个节点,叶子节点的个数为2^(k-1); - 具有
n个节点的满二叉树的深度为log_2^(n+1)。
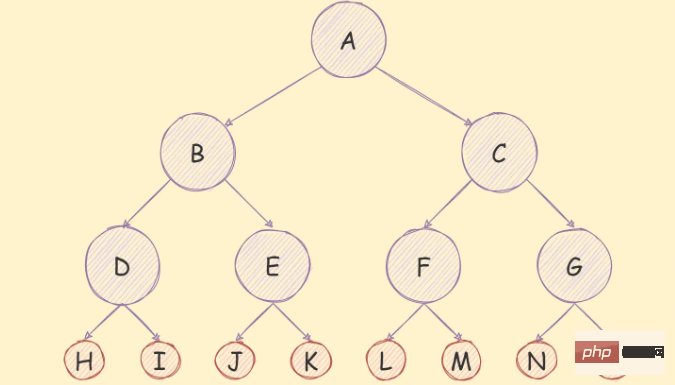
完全二叉树
如果一个二叉树去掉最后一次层是满二叉树,且最后一次的节点是依次从左到右分布的,则这个二叉树是一个完全二叉树,
如下图所示:
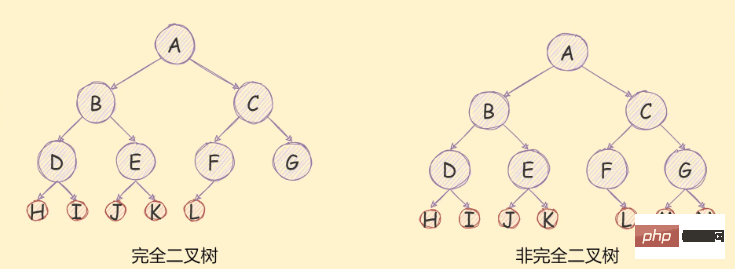
二叉树的存储
存储二叉树的常见方式分为两种,一种是使用数组存储,另一种使用链表存储。
数组存储
使用数组存储二叉树,如果遇到完全二叉树,存储顺序从上到下,从左到右,如下图所示:
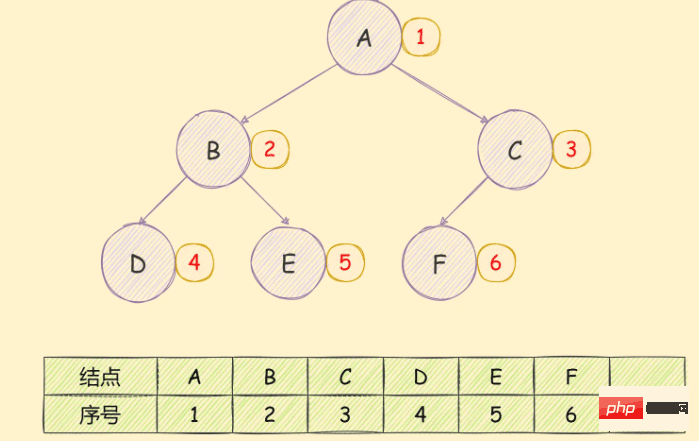
如果是一个非完全二叉树,如下图所示:
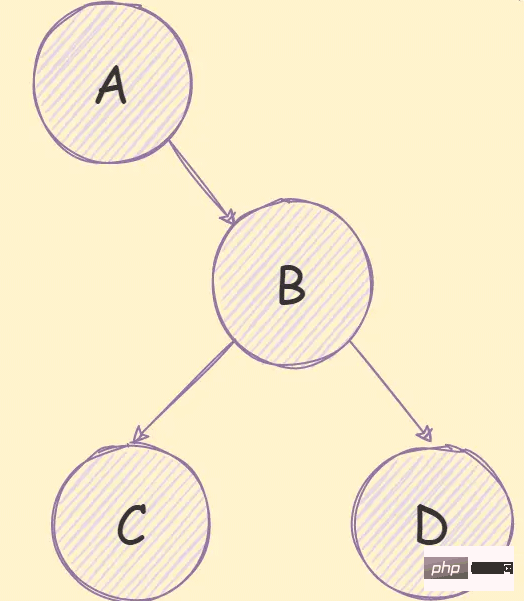
需要先将其转换为完全二叉树,然后在进行存储,如下图所示:
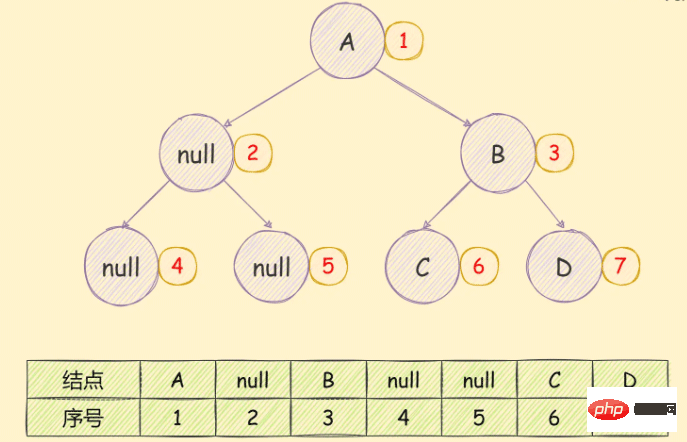
可以很明显的看到存储空间的浪费。
链表存储
使用链表存储通常将二叉树中的分为3个部分,如下图:

这三个部分依次是左子树的引用,该节点包含的数据,右子树的引用,存储方式如下图所示:
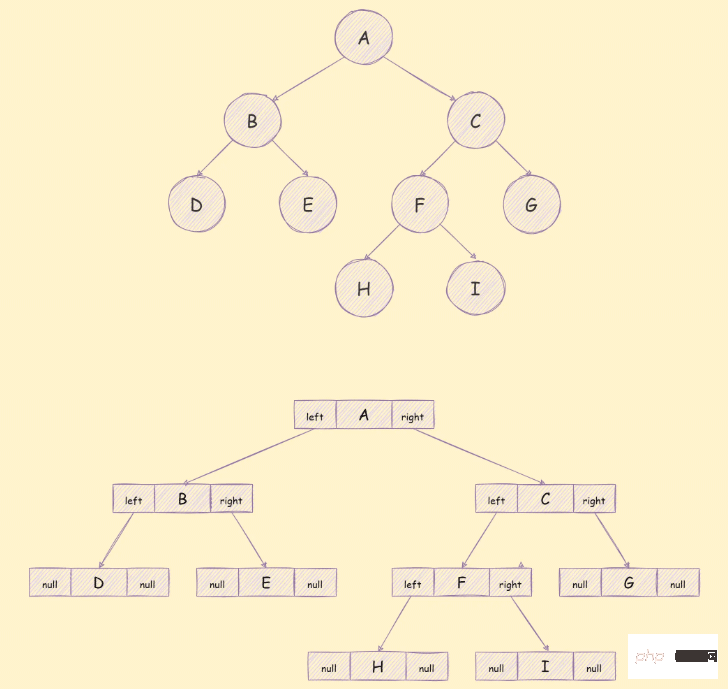
与二叉树相关的算法
以下算法中遍历用到的树如下:
// tree.js
const bt = {
val: 'A',
left: {
val: 'B',
left: { val: 'D', left: null, right: null },
right: { val: 'E', left: null, right: null },
},
right: {
val: 'C',
left: {
val: 'F',
left: { val: 'H', left: null, right: null },
right: { val: 'I', left: null, right: null },
},
right: { val: 'G', left: null, right: null },
},
}
module.exports = bt深度优先遍历
二叉树的深度优先遍历与树的深度优先遍历思路一致,思路如下:
- 访问根节点;
- 访问根节点的
left - 访问根节点的
right - 重复执行第二三步
实现代码如下:
const bt = {
val: 'A',
left: {
val: 'B',
left: { val: 'D', left: null, right: null },
right: { val: 'E', left: null, right: null },
},
right: {
val: 'C',
left: {
val: 'F',
left: { val: 'H', left: null, right: null },
right: { val: 'I', left: null, right: null },
},
right: { val: 'G', left: null, right: null },
},
}
function dfs(root) {
if (!root) return
console.log(root.val)
root.left && dfs(root.left)
root.right && dfs(root.right)
}
dfs(bt)
/** 结果
A B D E C F H I G
*/广度优先遍历
实现思路如下:
- 创建队列,把根节点入队
- 把对头出队并访问
- 把队头的
left和right依次入队 - 重复执行2、3步,直到队列为空
实现代码如下:
function bfs(root) {
if (!root) return
const queue = [root]
while (queue.length) {
const node = queue.shift()
console.log(node.val)
node.left && queue.push(node.left)
node.right && queue.push(node.right)
}
}
bfs(bt)
/** 结果
A B C D E F G H I
*/先序遍历
二叉树的先序遍历实现思想如下:
- 访问根节点;
- 对当前节点的左子树进行先序遍历;
- 对当前节点的右子树进行先序遍历;
如下图所示:

递归方式实现如下:
const bt = require('./tree')
function preorder(root) {
if (!root) return
console.log(root.val)
preorder(root.left)
preorder(root.right)
}
preorder(bt)
/** 结果
A B D E C F H I G
*/迭代方式实现如下:
// 非递归版
function preorder(root) {
if (!root) return
// 定义一个栈,用于存储数据
const stack = [root]
while (stack.length) {
const node = stack.pop()
console.log(node.val)
/* 由于栈存在先入后出的特性,所以需要先入右子树才能保证先出左子树 */
node.right && stack.push(node.right)
node.left && stack.push(node.left)
}
}
preorder(bt)
/** 结果
A B D E C F H I G
*/中序遍历
二叉树的中序遍历实现思想如下:
- 对当前节点的左子树进行中序遍历;
- 访问根节点;
- 对当前节点的右子树进行中序遍历;
如下图所示:
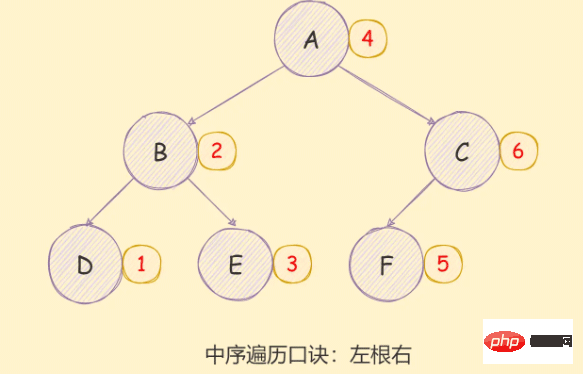
递归方式实现如下:
const bt = require('./tree')
// 递归版
function inorder(root) {
if (!root) return
inorder(root.left)
console.log(root.val)
inorder(root.right)
}
inorder(bt)
/** 结果
D B E A H F I C G
*/迭代方式实现如下:
// 非递归版
function inorder(root) {
if (!root) return
const stack = []
// 定义一个指针
let p = root
// 如果栈中有数据或者p不是null,则继续遍历
while (stack.length || p) {
// 如果p存在则一致将p入栈并移动指针
while (p) {
// 将 p 入栈,并以移动指针
stack.push(p)
p = p.left
}
const node = stack.pop()
console.log(node.val)
p = node.right
}
}
inorder(bt)
/** 结果
D B E A H F I C G
*/后序遍历
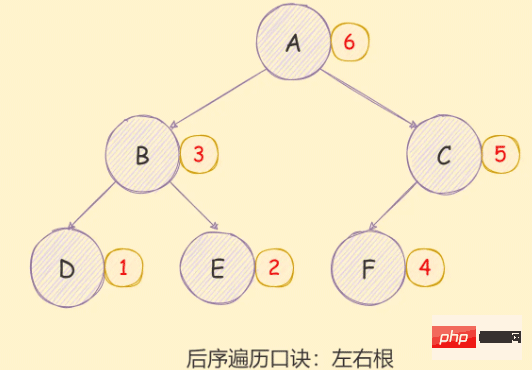
二叉树的后序遍历实现思想如下:
- 对当前节点的左子树进行后序遍历;
- 对当前节点的右子树进行后序遍历;
- 访问根节点;
如下图所示:
递归方式实现如下:
const bt = require('./tree')
// 递归版
function postorder(root) {
if (!root) return
postorder(root.left)
postorder(root.right)
console.log(root.val)
}
postorder(bt)
/** 结果
D E B H I F G C A
*/迭代方式实现如下:
// 非递归版
function postorder(root) {
if (!root) return
const outputStack = []
const stack = [root]
while (stack.length) {
const node = stack.pop()
outputStack.push(node)
// 这里先入left需要保证left后出,在stack中后出,就是在outputStack栈中先出
node.left && stack.push(node.left)
node.right && stack.push(node.right)
}
while (outputStack.length) {
const node = outputStack.pop()
console.log(node.val)
}
}
postorder(bt)
/** 结果
D E B H I F G C A
*/【相关推荐:javascript视频教程、web前端】
以上是详细介绍JavaScript二叉树及各种遍历算法的详细内容。更多信息请关注PHP中文网其他相关文章!
 JavaScript是用C编写的吗?检查证据Apr 25, 2025 am 12:15 AM
JavaScript是用C编写的吗?检查证据Apr 25, 2025 am 12:15 AM是的,JavaScript的引擎核心是用C语言编写的。1)C语言提供了高效性能和底层控制,适合JavaScript引擎的开发。2)以V8引擎为例,其核心用C 编写,结合了C的效率和面向对象特性。3)JavaScript引擎的工作原理包括解析、编译和执行,C语言在这些过程中发挥关键作用。
 JavaScript的角色:使网络交互和动态Apr 24, 2025 am 12:12 AM
JavaScript的角色:使网络交互和动态Apr 24, 2025 am 12:12 AMJavaScript是现代网站的核心,因为它增强了网页的交互性和动态性。1)它允许在不刷新页面的情况下改变内容,2)通过DOMAPI操作网页,3)支持复杂的交互效果如动画和拖放,4)优化性能和最佳实践提高用户体验。
 C和JavaScript:连接解释Apr 23, 2025 am 12:07 AM
C和JavaScript:连接解释Apr 23, 2025 am 12:07 AMC 和JavaScript通过WebAssembly实现互操作性。1)C 代码编译成WebAssembly模块,引入到JavaScript环境中,增强计算能力。2)在游戏开发中,C 处理物理引擎和图形渲染,JavaScript负责游戏逻辑和用户界面。
 从网站到应用程序:JavaScript的不同应用Apr 22, 2025 am 12:02 AM
从网站到应用程序:JavaScript的不同应用Apr 22, 2025 am 12:02 AMJavaScript在网站、移动应用、桌面应用和服务器端编程中均有广泛应用。1)在网站开发中,JavaScript与HTML、CSS一起操作DOM,实现动态效果,并支持如jQuery、React等框架。2)通过ReactNative和Ionic,JavaScript用于开发跨平台移动应用。3)Electron框架使JavaScript能构建桌面应用。4)Node.js让JavaScript在服务器端运行,支持高并发请求。
 Python vs. JavaScript:比较用例和应用程序Apr 21, 2025 am 12:01 AM
Python vs. JavaScript:比较用例和应用程序Apr 21, 2025 am 12:01 AMPython更适合数据科学和自动化,JavaScript更适合前端和全栈开发。1.Python在数据科学和机器学习中表现出色,使用NumPy、Pandas等库进行数据处理和建模。2.Python在自动化和脚本编写方面简洁高效。3.JavaScript在前端开发中不可或缺,用于构建动态网页和单页面应用。4.JavaScript通过Node.js在后端开发中发挥作用,支持全栈开发。
 C/C在JavaScript口译员和编译器中的作用Apr 20, 2025 am 12:01 AM
C/C在JavaScript口译员和编译器中的作用Apr 20, 2025 am 12:01 AMC和C 在JavaScript引擎中扮演了至关重要的角色,主要用于实现解释器和JIT编译器。 1)C 用于解析JavaScript源码并生成抽象语法树。 2)C 负责生成和执行字节码。 3)C 实现JIT编译器,在运行时优化和编译热点代码,显着提高JavaScript的执行效率。
 JavaScript在行动中:现实世界中的示例和项目Apr 19, 2025 am 12:13 AM
JavaScript在行动中:现实世界中的示例和项目Apr 19, 2025 am 12:13 AMJavaScript在现实世界中的应用包括前端和后端开发。1)通过构建TODO列表应用展示前端应用,涉及DOM操作和事件处理。2)通过Node.js和Express构建RESTfulAPI展示后端应用。
 JavaScript和Web:核心功能和用例Apr 18, 2025 am 12:19 AM
JavaScript和Web:核心功能和用例Apr 18, 2025 am 12:19 AMJavaScript在Web开发中的主要用途包括客户端交互、表单验证和异步通信。1)通过DOM操作实现动态内容更新和用户交互;2)在用户提交数据前进行客户端验证,提高用户体验;3)通过AJAX技术实现与服务器的无刷新通信。


热AI工具

Undresser.AI Undress
人工智能驱动的应用程序,用于创建逼真的裸体照片

AI Clothes Remover
用于从照片中去除衣服的在线人工智能工具。

Undress AI Tool
免费脱衣服图片

Clothoff.io
AI脱衣机

Video Face Swap
使用我们完全免费的人工智能换脸工具轻松在任何视频中换脸!

热门文章

热工具

PhpStorm Mac 版本
最新(2018.2.1 )专业的PHP集成开发工具

Atom编辑器mac版下载
最流行的的开源编辑器

WebStorm Mac版
好用的JavaScript开发工具

SecLists
SecLists是最终安全测试人员的伙伴。它是一个包含各种类型列表的集合,这些列表在安全评估过程中经常使用,都在一个地方。SecLists通过方便地提供安全测试人员可能需要的所有列表,帮助提高安全测试的效率和生产力。列表类型包括用户名、密码、URL、模糊测试有效载荷、敏感数据模式、Web shell等等。测试人员只需将此存储库拉到新的测试机上,他就可以访问到所需的每种类型的列表。

EditPlus 中文破解版
体积小,语法高亮,不支持代码提示功能






