使用回溯法解决八皇后问题

八皇后问题是找到一个解决方案，在棋盘的每一行放置一个皇后，使得两个皇后不能互相攻击。该问题可以使用递归来解决。在本节中，我们将介绍一种常见的算法设计技术，称为回溯来解决这个问题。回溯方法逐步搜索候选解决方案，一旦确定
就放弃该选项 候选方案不可能是有效的解决方案，然后寻找新的候选方案。

可以使用二维数组来表示棋盘。然而，由于每一行只能有一个皇后，因此使用一维数组来表示皇后在该行中的位置就足够了。因此，您可以将 queens 数组定义为：

int[] queens = new int[8];

将 j 分配给 queens[i] 表示皇后放置在行 i 和列 j 中。下图(a)显示了下图(b)中棋盘的queens数组的内容。

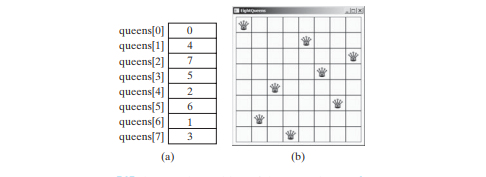
搜索从第一行开始，其中 k = 0，其中 k 是正在考虑的当前行的索引。该算法检查是否可以按 _j = 0, 1, ... , 7 的顺序将皇后放置在行中的第 j_ 列中。搜索实现如下：

• 如果成功，它会继续在下一行中搜索皇后的位置。如果当前行是最后一行，则找到解决方案。
• 如果不成功，则回溯到上一行并继续在上一行的下一列中搜索新的展示位置。
• 如果算法回溯到第一行并且无法在该行中找到皇后的新位置，则无法找到解决方案。

下面的代码给出了显示八皇后问题解决方案的程序。

package application;
import javafx.application.Application;
import javafx.geometry.Pos;
import javafx.stage.Stage;
import javafx.scene.Scene;
import javafx.scene.control.Label;
import javafx.scene.image.Image;
import javafx.scene.image.ImageView;
import javafx.scene.layout.GridPane;

public class EightQueens extends Application {
    public static final int SIZE = 8; // The size of the chess board
    // queens are placed at (i, queens[i])
    // -1 indicates that no queen is currently placed in the ith row
    // Initially, place a queen at (0, 0) in the 0th row
    private int[] queens = {-1, -1, -1, -1, -1, -1, -1, -1};

    @Override // Override the start method in the Application class
    public void start(Stage primaryStage) {
        search(); // Search for a solution

        // Display chess board
        GridPane chessBoard = new GridPane();
        chessBoard.setAlignment(Pos.CENTER);
        Label[][] labels = new Label[SIZE][SIZE];
        for(int i = 0; i < SIZE; i++)
            for(int j = 0; j < SIZE; j++) {
                chessBoard.add(labels[i][j] = new Label(), i, j);
                labels[i][j].setStyle("-fx-border-color: black");
                labels[i][j].setPrefSize(55, 55);
            }

        // Display queens
        Image image = new Image("file:C:/Users/Paul/development/MyJavaFX/src/application/image/lo.jpg");
        for(int i = 0; i < SIZE; i++)
            labels[i][queens[i]].setGraphic(new ImageView(image));

        // Create a scene and place it in the stage
        Scene scene = new Scene(chessBoard, 55 * SIZE, 55 * SIZE);
        primaryStage.setTitle("EightQueens"); // Set the stage title
        primaryStage.setScene(scene); // Place the scene in the stage
        primaryStage.show(); // Display the stage
    }

    public static void main(String[] args) {
        Application.launch(args);
    }

    /** Search for a solution */
    private boolean search() {
        // k - 1 indicates the number of queens placed so far
        // We are looking for a position in the kth row to place a queen
        int k = 0;
        while(k >= 0 && k < SIZE) {
            // Find a position to place a queen in the kth row
            int j = findPosition(k);
            if(j < 0) {
                queens[k] = -1;
                k--; // back track to the previous row
            } else {
                queens[k] = j;
                k++;
            }
        }

        if(k == -1)
            return false; // No solution
        else
            return true; // A solution is found
    }

    public int findPosition(int k) {
        int start = queens[k] + 1; // Search for a new placement

        for(int j =start; j < SIZE; j++) {
            if(isValid(k, j))
                return j; // (k, j) is the place to put the queen now
        }

        return -1;
    }

    /** Return true is a queen can be placed at (row, column) */
    public boolean isValid(int row, int column) {
        for(int i = 1; i <= row; i++)
            if(queens[row - i] == column // Check column
            || queens[row - i] == column - i // Check upleft diagonal
            || queens[row - i] == column + i) // Check upright diagonal
                return false; // There is a conflict
        return true; // No conflict
    }

}

程序调用search()（第20行）来搜索解决方案。最初，任何行中都没有放置皇后（第 16 行）。现在，搜索从第一行 k = 0（第 53 行）开始，并找到皇后的位置（第 56 行）。如果成功，将其放入该行（第 61 行）并考虑下一行（第 62 行）。如果不成功，则回溯到上一行（第 58-59 行）。

findPosition(k) 方法从 queen[k] + 1 开始搜索在行 k 中放置皇后的可能位置（第 73 行） ）。它检查是否可以将皇后放置在 start, start + 1, 。 。 。 、和 7，按此顺序（第 75-78 行）。如果可能，返回列索引（第77行）；否则，返回 -1（第 80 行）。

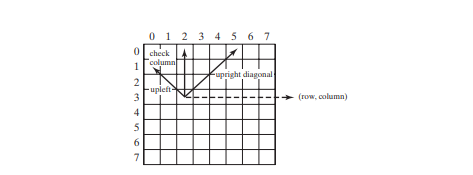
调用isValid(row, column)方法来检查在指定位置放置皇后是否会与之前放置的皇后发生冲突（第76行）。它确保没有皇后被放置在同一列（第86行）、左上角对角线（第87行）或右上角对角线（第88行），如下图所示。

以上是使用回溯法解决八皇后问题的详细内容。更多信息请关注PHP中文网其他相关文章！