C程序中的Peterson圖表問題

假設我們有一個如下所示的圖形。這個圖形是彼得森圖。頂點從0到9編號。每個頂點都有一些字母。考慮一個在該圖中的行走W，其中使用了L個頂點。當行走W中的字母序列和S相同時，字串S由行走W實現。我們可以多次訪問頂點。

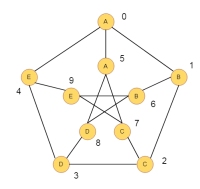
例如，一個字串S類似於“ABBECCD”，這由行走(0, 1, 6, 9, 7, 2, 3)實現。我們的任務是找到這樣的行走，如果存在這樣的行走，則找到字典順序最小的行走。如果沒有這樣的行走，則回傳-1。

演算法

petersonGraphWalk(S, v) -

begin
   res := starting vertex
   for each character c in S except the first one, do
      if there is an edge between v and c in outer graph, then      
         v := c
      else if there is an edge between v and c+5 in inner graph, then
         v := c + 5
      else
         return false
      end if
         put v into res
      done
   return true
end

Example

的中文翻譯為：

範例

#include<iostream>
using namespace std;
bool adj_mat[10][10] = {{0, 1, 0, 0, 1, 1, 0, 0, 0, 0},
   {1, 0, 1, 0, 0, 0, 1, 0, 0, 0},
   {0, 1, 0, 1, 0, 0, 0, 1, 0, 0},
   {0, 0, 1, 0, 1, 0, 0, 0, 1, 0},
   {1, 0, 0, 1, 0, 0, 0, 0, 0, 1},
   {1, 0, 0, 0, 0, 0, 0, 1, 1, 0},
   {0, 1, 0, 0, 0, 0, 0, 0, 1, 1},
   {0, 0, 1, 0, 0, 1, 0, 0, 0, 1},
   {0, 0, 0, 1, 0, 1, 1, 0, 0, 0},
   {0, 0, 0, 0, 1, 0, 1, 1, 0, 0}
};
char S[100005];
char res[100005];
bool petersonGraphWalk(char* S, int v){
   res[0] = v + &#39;0&#39;;
   for(int i = 1; S[i]; i++){
      //traverse the outer graph
      if(adj_mat[v][S[i] - &#39;A&#39;] || adj_mat[S[i] - &#39;A&#39;][v]){
         v = S[i] - &#39;A&#39;;
      }
      //then check the inner graph
      else if(adj_mat[v][S[i] - &#39;A&#39; + 5] || adj_mat[S[i] - &#39;A&#39; + 5][v]){
         v = S[i] - &#39;A&#39; + 5;
      }else{
         return false;
      }
      res[i] = v + &#39;0&#39;;
   }
   return true;
}
main() {
   char* str = "ABBECCD";
   if(petersonGraphWalk(str, str[0] - &#39;A&#39;) || petersonGraphWalk(str, str[0] - &#39;A&#39; + 5)){
      cout << res;
   }else{
      cout << -1;
   }
}

#輸出

0169723

以上是C程序中的Peterson圖表問題的詳細內容。更多資訊請關注PHP中文網其他相關文章！