물리학에서 이중 스프링 질량 에너지 시스템을 해결하기 위한 Python 코드 예제
- 黄舟원래의
- 2017-10-03 05:46:181881검색
이 글은 물리학에서 이중 스프링 질량 에너지 시스템을 해결하기 위해 Python을 사용하는 방법에 대한 관련 정보를 주로 소개합니다. 이 글은 모든 사람의 학습이나 작업에 대한 특정 참고 학습 가치를 제공합니다. 친구가 필요하고, 편집자를 따라가서 함께 배워보세요.
머리말
이 글은 물리학에서 이중 스프링 질량 에너지 시스템을 해결하기 위해 Python을 사용하는 것과 관련된 내용을 주로 소개하며, 참고 및 연구를 위해 공유합니다. 아래에서는 많은 말을 하지 않겠습니다. , 세부 사항을 살펴 보겠습니다.
물리적 모델은 다음과 같습니다:
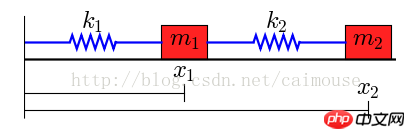
이 시스템에는 두 개의 물체가 있으며, 질량은 각각 m1과 m2이고 두 개의 스프링으로 서로 연결되어 있으며 망원경 시스템은 k1과 k2이며 왼쪽 끝입니다. 결정된. 외력이 없다고 가정하면 두 스프링의 길이는 L1과 L2입니다.
두 물체에는 중력이 있으므로 평면에 마찰이 생기고 마찰계수는 각각 b1과 b2가 됩니다. 따라서 다음과 같이 미분방정식을 작성할 수 있습니다.

이것은 2차 미분방정식입니다. 이를 Python을 사용하여 풀려면 1차 미분방정식으로 변환해야 합니다. 따라서 다음 두 가지 변수가 도입됩니다.

이 두 변수는 이동 속도와 동일합니다. 연산을 통해 이렇게 바꿀 수 있습니다:
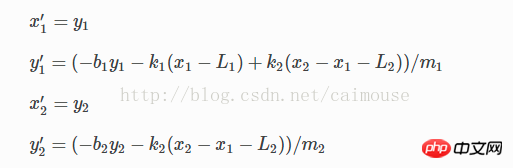
이때 선형방정식을 벡터배열로 바꿀 수 있고, 파이썬을 이용해 정의할 수 있습니다.
코드는 다음과 같습니다.
# Use ODEINT to solve the differential equations defined by the vector field
from scipy.integrate import odeint
def vectorfield(w, t, p):
"""
Defines the differential equations for the coupled spring-mass system.
Arguments:
w : vector of the state variables:
w = [x1,y1,x2,y2]
t : time
p : vector of the parameters:
p = [m1,m2,k1,k2,L1,L2,b1,b2]
"""
x1, y1, x2, y2 = w
m1, m2, k1, k2, L1, L2, b1, b2 = p
# Create f = (x1',y1',x2',y2'):
f = [y1,
(-b1 * y1 - k1 * (x1 - L1) + k2 * (x2 - x1 - L2)) / m1,
y2,
(-b2 * y2 - k2 * (x2 - x1 - L2)) / m2]
return f
# Parameter values
# Masses:
m1 = 1.0
m2 = 1.5
# Spring constants
k1 = 8.0
k2 = 40.0
# Natural lengths
L1 = 0.5
L2 = 1.0
# Friction coefficients
b1 = 0.8
b2 = 0.5
# Initial conditions
# x1 and x2 are the initial displacements; y1 and y2 are the initial velocities
x1 = 0.5
y1 = 0.0
x2 = 2.25
y2 = 0.0
# ODE solver parameters
abserr = 1.0e-8
relerr = 1.0e-6
stoptime = 10.0
numpoints = 250
# Create the time samples for the output of the ODE solver.
# I use a large number of points, only because I want to make
# a plot of the solution that looks nice.
t = [stoptime * float(i) / (numpoints - 1) for i in range(numpoints)]
# Pack up the parameters and initial conditions:
p = [m1, m2, k1, k2, L1, L2, b1, b2]
w0 = [x1, y1, x2, y2]
# Call the ODE solver.
wsol = odeint(vectorfield, w0, t, args=(p,),
atol=abserr, rtol=relerr)
with open('two_springs.dat', 'w') as f:
# Print & save the solution.
for t1, w1 in zip(t, wsol):
out = '{0} {1} {2} {3} {4}\n'.format(t1, w1[0], w1[1], w1[2], w1[3]);
print(out)
f.write(out);여기에 결과를 출력합니다. two_springs.dat 파일로 가서 데이터를 그림으로 표시하는 프로그램을 작성하면 코드는 다음과 같습니다.
# Plot the solution that was generated from numpy import loadtxt from pylab import figure, plot, xlabel, grid, hold, legend, title, savefig from matplotlib.font_manager import FontProperties t, x1, xy, x2, y2 = loadtxt('two_springs.dat', unpack=True) figure(1, figsize=(6, 4.5)) xlabel('t') grid(True) lw = 1 plot(t, x1, 'b', linewidth=lw) plot(t, x2, 'g', linewidth=lw) legend((r'$x_1$', r'$x_2$'), prop=FontProperties(size=16)) title('Mass Displacements for the\nCoupled Spring-Mass System') savefig('two_springs.png', dpi=100)
마지막으로, 다음과 같이 출력된 png 그림을 확인해 보겠습니다.
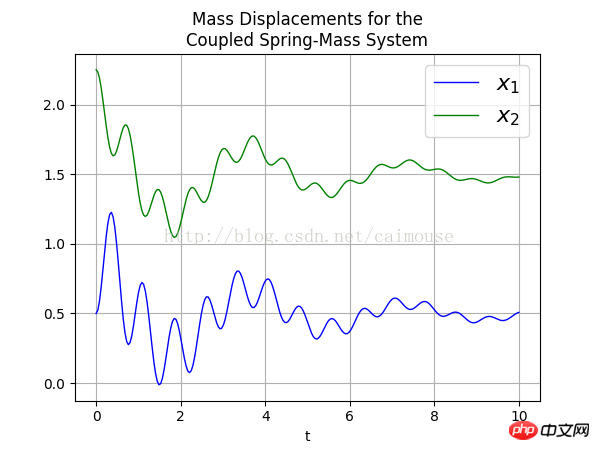
요약
위 내용은 물리학에서 이중 스프링 질량 에너지 시스템을 해결하기 위한 Python 코드 예제의 상세 내용입니다. 자세한 내용은 PHP 중국어 웹사이트의 기타 관련 기사를 참조하세요!

