Application of Baum-Welch algorithm in implicit Markov model

The Hidden Markov Model (HMM) is a commonly used statistical model for modeling and forecasting time series data. The Baum-Welch algorithm, also known as the forward-backward algorithm, is an unsupervised learning algorithm used for HMM parameter estimation. This article will introduce the principle and implementation process of the Baum-Welch algorithm in detail.
1. Introduction to HMM
Before introducing the Baum-Welch algorithm, let’s first understand the HMM model. The HMM model is a probabilistic model used to describe the process of randomly generating observation sequences by hidden Markov chains. A hidden Markov chain consists of a set of states and transition probabilities between states, and the observation sequence consists of the observations generated by each state. The basic assumption of the HMM model is that each observation value in the observation sequence only depends on the current state and has nothing to do with past states and observations. The Baum-Welch algorithm is an unsupervised learning algorithm used to estimate the parameters of HMM models. It iteratively adjusts the transition probability and emission probability of the model according to the observation sequence, so that the model can better fit the observation data. Through multiple iterations, the Baum-Welch algorithm can find the optimal model parameters, thereby more accurately describing the generation process of the observation sequence.
The HMM model can be described by three parameters:
1. Initial state probability vector (π), representing the initial state probability of the model ;
2. State transition probability matrix (A), indicating the probability of transitioning from one state to another;
3. Observation Probability matrix (B), representing the probability of generating an observation in each state.
HMM models usually use forward and backward algorithms for prediction and inference. However, three parameters in the HMM model need to be estimated from training data. This is what the Baum-Welch algorithm does.
2. Principle of Baum-Welch algorithm
The Baum-Welch algorithm is an unsupervised learning algorithm based on the EM algorithm, which is used to Three parameters of the HMM model are estimated. The EM algorithm is an iterative algorithm that maximizes the likelihood function to solve parameters by alternating E-steps and M-steps. In HMM, the E step calculates the probability of being in each state at each moment given the current parameters; the M step updates the model parameters through these probabilities.
Specifically, the process of the Baum-Welch algorithm is as follows:
1. Randomly initialize the model parameters (π, A, B);
2. Use the forward algorithm and the backward algorithm to calculate the probability of being in each state at each moment given the current parameters;
3. Use these probabilities to update the model parameters, specifically, update the initial state probability vector π, state transition probability matrix A and observation probability matrix B;
4. Repeat steps 2 and Step 3 until the model parameters converge.
In step E, we need to calculate the probability of being in each state at each moment given the current parameters. Specifically, we need to calculate the forward probability α and backward probability β:
α_t(i)=P(O_1,O_2,…,O_t,q_t=i|λ)
β_t(i)=P(O_t 1,O_t 2,…,O_T|q_t=i,λ)
where, λ represents the current model parameters, O represents the observation sequence, and q represents the state sequence. α_t(i) represents the probability of being in state i at time t, and β_t(i) represents the probability of the observation sequence from time t 1 to time T, given the condition of state i. α and β can be calculated recursively.
In step M, we need to use these probabilities to update the model parameters. Specifically, we need to calculate the new initial state probability vector π, state transition probability matrix A and observation probability matrix B:
π_i=α_1(i)β_1(i)/P (O|λ)
A_ij=∑_(t=1)^(T-1)α_t(i)a_ij b_j(O_t 1)β_t 1(j)/∑_ (t=1)^(T-1)α_t(i)β_t(i)
B_j(k)=∑_(t=1)^(T-1)γ_t (j,k)/∑_(t=1)^(T-1)γ_t(j)
where, γ_t(i,j) means that it is in state i at time t And the probability that 1 is in state j at time t 1, P(O|λ) represents the probability of the observation sequence. You can use these formulas to update model parameters.
The convergence of the Baum-Welch algorithm is guaranteed, but it may converge to a local optimal solution. In order to avoid this situation, it is usually necessary to run the Baum-Welch algorithm multiple times and select the optimal model parameters.
3. Implementation of Baum-Welch algorithm
The implementation of Baum-Welch algorithm usually involves some technical details. The following are some implementation details of the Baum-Welch algorithm:
1. Avoid numerical underflow
When calculating α and β, due to the probability The value is very small and numerical underflow may occur. To avoid this, the log probability and log likelihood functions can be used for calculations.
2. Avoid zero probability
When calculating B, a certain state may generate a certain observation at a certain point in time The probability of the value is zero. To avoid this, you can use smoothing techniques such as additive smoothing or multiplicative smoothing.
3. Use multiple runs
Since the Baum-Welch algorithm may converge to a local optimal solution, multiple runs are usually required algorithm and select the optimal model parameters.
In general, the Baum-Welch algorithm is an unsupervised learning algorithm based on the EM algorithm and is widely used in natural language processing, speech recognition and other fields.
The above is the detailed content of Application of Baum-Welch algorithm in implicit Markov model. For more information, please follow other related articles on the PHP Chinese website!
 Handling NULL Values in SQLApr 11, 2025 am 09:37 AM
Handling NULL Values in SQLApr 11, 2025 am 09:37 AMIntroduction In the realm of databases, NULL values often present unique challenges. Representing missing, undefined, or unknown data, they can complicate data management and analysis. Consider a sales database with missing customer feedback or orde
 How to Integrate Google Gemini into Tableau Dashboards?Apr 11, 2025 am 09:27 AM
How to Integrate Google Gemini into Tableau Dashboards?Apr 11, 2025 am 09:27 AMHarnessing the Power of Google Gemini in Tableau Dashboards: An AI-Powered Enhancement Tableau's robust visualization capabilities, spanning data preparation (Tableau Prep Builder), data storytelling (Tableau Desktop), and collaborative sharing (Tabl
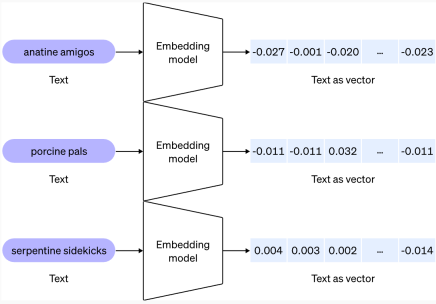 What are Vector Embeddings? Types and Use CasesApr 11, 2025 am 09:18 AM
What are Vector Embeddings? Types and Use CasesApr 11, 2025 am 09:18 AMUnlocking the Power of Vector Embeddings: A Guide to Generative AI Imagine explaining RAG (Retrieval Augmented Generation) to someone who doesn't speak your language – a daunting task, right? Now consider machines, which also struggle to "under
 Nested Queries in SQLApr 11, 2025 am 09:17 AM
Nested Queries in SQLApr 11, 2025 am 09:17 AMIntroduction Imagine searching a vast library containing books with nested books within. To find specific information, you might need to consult the smaller books first, then use that information to locate the larger one. This illustrates the concep
 Understanding SQL WHERE ClauseApr 11, 2025 am 09:07 AM
Understanding SQL WHERE ClauseApr 11, 2025 am 09:07 AMSQL WHERE Clause: A Comprehensive Guide The SQL WHERE clause is a fundamental component of SQL statements, used for filtering records and retrieving specific data from a database. Imagine a vast customer database – the WHERE clause allows you to pin
 Newest Annual Compilation Of The Best Prompt Engineering TechniquesApr 10, 2025 am 11:22 AM
Newest Annual Compilation Of The Best Prompt Engineering TechniquesApr 10, 2025 am 11:22 AMFor those of you who might be new to my column, I broadly explore the latest advances in AI across the board, including topics such as embodied AI, AI reasoning, high-tech breakthroughs in AI, prompt engineering, training of AI, fielding of AI, AI re
 Europe's AI Continent Action Plan: Gigafactories, Data Labs, And Green AIApr 10, 2025 am 11:21 AM
Europe's AI Continent Action Plan: Gigafactories, Data Labs, And Green AIApr 10, 2025 am 11:21 AMEurope's ambitious AI Continent Action Plan aims to establish the EU as a global leader in artificial intelligence. A key element is the creation of a network of AI gigafactories, each housing around 100,000 advanced AI chips – four times the capaci
 Is Microsoft's Straightforward Agent Story Enough To Create More Fans?Apr 10, 2025 am 11:20 AM
Is Microsoft's Straightforward Agent Story Enough To Create More Fans?Apr 10, 2025 am 11:20 AMMicrosoft's Unified Approach to AI Agent Applications: A Clear Win for Businesses Microsoft's recent announcement regarding new AI agent capabilities impressed with its clear and unified presentation. Unlike many tech announcements bogged down in te


Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

AI Hentai Generator
Generate AI Hentai for free.

Hot Article

Hot Tools

SublimeText3 Linux new version
SublimeText3 Linux latest version

Zend Studio 13.0.1
Powerful PHP integrated development environment

SublimeText3 Chinese version
Chinese version, very easy to use

VSCode Windows 64-bit Download
A free and powerful IDE editor launched by Microsoft

mPDF
mPDF is a PHP library that can generate PDF files from UTF-8 encoded HTML. The original author, Ian Back, wrote mPDF to output PDF files "on the fly" from his website and handle different languages. It is slower than original scripts like HTML2FPDF and produces larger files when using Unicode fonts, but supports CSS styles etc. and has a lot of enhancements. Supports almost all languages, including RTL (Arabic and Hebrew) and CJK (Chinese, Japanese and Korean). Supports nested block-level elements (such as P, DIV),






