 Technology peripherals
Technology peripherals AI
AI Terence Tao's Crazy Amway Copilot: It helped me complete a one-page proof and even guessed my subsequent process
Terence Tao's Crazy Amway Copilot: It helped me complete a one-page proof and even guessed my subsequent processTerence Tao's Crazy Amway Copilot: It helped me complete a one-page proof and even guessed my subsequent process
After being “endorsed” by GPT-4, Copilot was also ambushed by Terence Tao.
He bluntly said that when programming, Copilot can directly predict what he will do next.
With Copilot, research has become more convenient, and Tao Zhexuan also used it to assist him in completing his latest research results.

Tao Zhexuan said that this part of the paper actually only has one page.
But to complete this one-page proof, he wrote more than 200 lines of code, using the newly learned programming language Lean4.

The GitHub page of Tao Zhexuan’s public code shows that Copilot has increased the speed of writing code by more than half.
Tao Zhexuan said that the reason why he chose Lean4 was because of its "rewriting strategy", which is to perform targeted partial replacement of a long expression.
For example, if a complex function f(x) is defined, when we want to enter the expression of f(114514), we can directly "rewrite" x into 114514 using code.
Tao Zhexuan said that this feature is not too convenient compared to LaTeX which requires repeated input of formulas.
So what new results has Tao Zhexuan’s “one-page proof” brought us this time?
One-page proof of new inequalities
This paper talks about issues related to MacLaughlin's inequality.
McLaughlin's inequality is a classic inequality in mathematics. It is derived based on the law that "the arithmetic mean of non-negative real numbers is greater than or equal to the geometric mean". It can be expressed as:
Assume y1…yn is a non-negative real number. For k=1…n, the mean Sk is defined as (the number of terms whose denominator is the numerator):
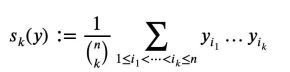
#It occurs as the normalized coefficient of a polynomial of degree n with roots.
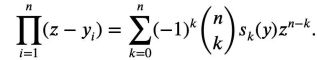
(Remember this formula, we call it Formula 1)
Then MacLaughlin’s inequality can be expressed as:
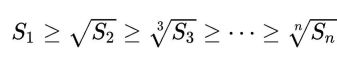
Among them, the equal sign holds true if and only if all yi are equal.
In calculus, there is also a classic Newton's inequality:

For any 1≤k
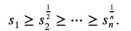
But if this restriction is not added, negative numbers are allowed With the existence of terms, Maclaurin's inequality cannot be expressed using Newton's inequality.
So for the situation where negative terms may exist in Newton's inequalities, Tao Zhexuan proposed a new set of inequality variants:
For any r>0 and 1≤ℓ≤n, there must be a formula 2 or Equation 3 is established.

This is what Terence Tao is trying to prove on this page. The specific proof process is as follows:
We might as well construct a polynomial about the complex variable z P(z):
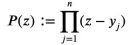
From the previous equation 1 and the triangle inequality, we can get:
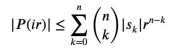
So we only need to establish the lower bound:
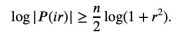
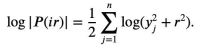
t a) is convex and a>0, we can get the inequality:

2, t=2log yj, it can be concluded:
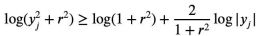
n|=1, the following formula holds:

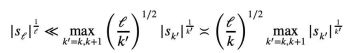

Paper address: https://arxiv.org/abs/2310.05328
The above is the detailed content of Terence Tao's Crazy Amway Copilot: It helped me complete a one-page proof and even guessed my subsequent process. For more information, please follow other related articles on the PHP Chinese website!
 An AI Space Company Is BornMay 12, 2025 am 11:07 AM
An AI Space Company Is BornMay 12, 2025 am 11:07 AMThis article showcases how AI is revolutionizing the space industry, using Tomorrow.io as a prime example. Unlike established space companies like SpaceX, which weren't built with AI at their core, Tomorrow.io is an AI-native company. Let's explore
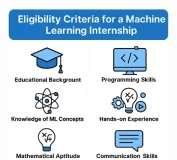 10 Machine Learning Internships in India (2025)May 12, 2025 am 10:47 AM
10 Machine Learning Internships in India (2025)May 12, 2025 am 10:47 AMLand Your Dream Machine Learning Internship in India (2025)! For students and early-career professionals, a machine learning internship is the perfect launchpad for a rewarding career. Indian companies across diverse sectors – from cutting-edge GenA
 Try Fellou AI and Say Goodbye to Google and ChatGPTMay 12, 2025 am 10:26 AM
Try Fellou AI and Say Goodbye to Google and ChatGPTMay 12, 2025 am 10:26 AMThe landscape of online browsing has undergone a significant transformation in the past year. This shift began with enhanced, personalized search results from platforms like Perplexity and Copilot, and accelerated with ChatGPT's integration of web s
 Personal Hacking Will Be A Pretty Fierce BearMay 11, 2025 am 11:09 AM
Personal Hacking Will Be A Pretty Fierce BearMay 11, 2025 am 11:09 AMCyberattacks are evolving. Gone are the days of generic phishing emails. The future of cybercrime is hyper-personalized, leveraging readily available online data and AI to craft highly targeted attacks. Imagine a scammer who knows your job, your f
 Pope Leo XIV Reveals How AI Influenced His Name ChoiceMay 11, 2025 am 11:07 AM
Pope Leo XIV Reveals How AI Influenced His Name ChoiceMay 11, 2025 am 11:07 AMIn his inaugural address to the College of Cardinals, Chicago-born Robert Francis Prevost, the newly elected Pope Leo XIV, discussed the influence of his namesake, Pope Leo XIII, whose papacy (1878-1903) coincided with the dawn of the automobile and
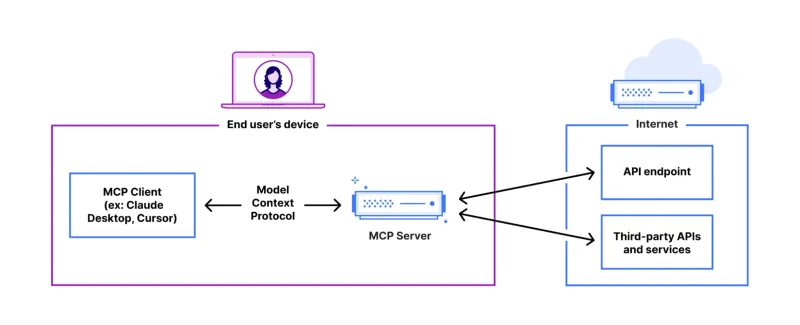 FastAPI-MCP Tutorial for Beginners and Experts - Analytics VidhyaMay 11, 2025 am 10:56 AM
FastAPI-MCP Tutorial for Beginners and Experts - Analytics VidhyaMay 11, 2025 am 10:56 AMThis tutorial demonstrates how to integrate your Large Language Model (LLM) with external tools using the Model Context Protocol (MCP) and FastAPI. We'll build a simple web application using FastAPI and convert it into an MCP server, enabling your L
 Dia-1.6B TTS : Best Text-to-Dialogue Generation Model - Analytics VidhyaMay 11, 2025 am 10:27 AM
Dia-1.6B TTS : Best Text-to-Dialogue Generation Model - Analytics VidhyaMay 11, 2025 am 10:27 AMExplore Dia-1.6B: A groundbreaking text-to-speech model developed by two undergraduates with zero funding! This 1.6 billion parameter model generates remarkably realistic speech, including nonverbal cues like laughter and sneezes. This article guide
 3 Ways AI Can Make Mentorship More Meaningful Than EverMay 10, 2025 am 11:17 AM
3 Ways AI Can Make Mentorship More Meaningful Than EverMay 10, 2025 am 11:17 AMI wholeheartedly agree. My success is inextricably linked to the guidance of my mentors. Their insights, particularly regarding business management, formed the bedrock of my beliefs and practices. This experience underscores my commitment to mentor


Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

SublimeText3 English version
Recommended: Win version, supports code prompts!

EditPlus Chinese cracked version
Small size, syntax highlighting, does not support code prompt function

ZendStudio 13.5.1 Mac
Powerful PHP integrated development environment

Safe Exam Browser
Safe Exam Browser is a secure browser environment for taking online exams securely. This software turns any computer into a secure workstation. It controls access to any utility and prevents students from using unauthorized resources.

VSCode Windows 64-bit Download
A free and powerful IDE editor launched by Microsoft






