Learn more about the double-ended diff algorithm in Vue

The diff algorithm is the most complex part of the renderer. This article will take you through the double-ended diff algorithm in Vue. I hope it will be helpful to you!

Vue and React are both front-end frameworks based on vdom. Component rendering will return vdom, and the renderer will synchronize vdom to dom through the addition, deletion and modification API. (Learning video sharing: vuejs video tutorial)

When rendering again, a new vdom will be generated. The renderer will compare the two vdom trees and add, delete, and modify the different parts. api updated to dom.

The algorithm that compares two vdom trees and finds the differences is called the diff algorithm.

The diff algorithm is the most complex part of the renderer and is also a hot question in interviews. Today we will explore the diff algorithm through Vue’s diff algorithm.

diff algorithm

We know that the complexity of diffing two trees is O(n^3), because each node has to communicate with another All nodes in the tree are compared once, which is n. If a changed node is found, the complexity of inserting, deleting, and modifying is also n. This is true for all nodes, multiplied by n, so the complexity is O(n * n * n).

Such complexity is unacceptable for the front-end framework, which means that 1000 nodes must be processed in one rendering, 1000 * 1000 * 1000, a total of 1 billion Second-rate.

So the diff of the front-end framework agrees on two processing principles: Only compare the same layer, and if the type changes, the child nodes will no longer be compared.

Because there are relatively few cases of cross-level movement of DOM nodes. Generally, it is the addition, deletion and modification of DOM at the same level.

In this way, you only need to traverse once and compare the types. The complexity is O(n). Moreover, if the type changes, the child nodes will no longer be compared, which can save the traversal of a large number of nodes. In addition, because the associated dom nodes are recorded in vdom, there is no need to traverse the addition, deletion or modification of dom, which is O(1). The overall diff algorithm complexity is O(n) complexity.

1000 nodes are rendered at a time and compared up to 1000 times. This complexity is within the acceptable range.

But although such an algorithm has low complexity, it still has problems.

For example, for a group of nodes, assuming there are 5, the type is ABCDE, and the next rendering will be EABCD. At this time, compare one by one and find that the type is different, and the 5 nodes will be re-rendered.

Moreover, according to the principle of different types, child nodes are no longer compared. If these nodes have child nodes, they will also be re-rendered.

dom operation is relatively slow, so although the algorithm complexity of diff is low, the re-rendering performance is not high.

So, in addition to considering its own time complexity, the diff algorithm also needs to consider a factor: the number of dom operations.

In the above example, ABCDE becomes EABCD. Obviously, you only need to move E, and there is no need to create a new element at all.

But how to compare and find out that the same node has moved?

Determine type? That won't work. There may be many nodes of the same type and they cannot be distinguished.

It is best to have a unique identifier for each node.

So when rendering a group of nodes, the front-end framework will ask the developer to specify the key, and use the key to determine whether some nodes have just moved, so that they can be reused directly.

In this way, when the diff algorithm processes the comparison of a group of nodes, it must optimize the algorithm again based on the key.

We will call the diff algorithm of two groups of nodes based on key multi-node diff algorithm, which is part of the diff algorithm of the entire vdom.

Next let’s learn the multi-node diff algorithm:

Simple diff

Assume that a set of nodes ABCD is rendered, and DCAB is rendered again. This How to deal with it?

The purpose of the multi-node diff algorithm is to reuse nodes as much as possible and replace creation by moving nodes.

So for each node in the new vnode array, we have to find out whether there is a corresponding key in the old vnode array. If there is, move it to the new position. If not, create a new one.

That's it:

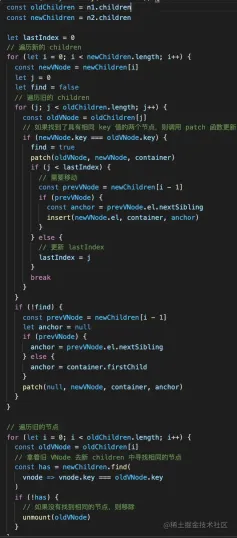
const oldChildren = n1.children
const newChildren = n2.children

let lastIndex = 0
// 遍历新的 children
for (let i = 0; i < newChildren.length; i++) {
    const newVNode = newChildren[i]
    let j = 0
    let find = false
    // 遍历旧的 children
    for (j; j < oldChildren.length; j++) {
      const oldVNode = oldChildren[j]
      // 如果找到了具有相同 key 值的两个节点，则调用 patch 函数更新
      if (newVNode.key === oldVNode.key) {
        find = true
        patch(oldVNode, newVNode, container)
        
        处理移动...
        
        break //跳出循环，处理下一个节点
      }
   }
   // 没有找到就是新增了
   if (!find) {
      const prevVNode = newChildren[i - 1]
      let anchor = null
      if (prevVNode) {
        anchor = prevVNode.el.nextSibling
      } else {
        anchor = container.firstChild
      }
      patch(null, newVNode, container, anchor)
   }
}

The patch function here is to update the properties of the node and reset the event listener. If there is no corresponding old node, the node is inserted, and a node after it needs to be passed in as the anchor anchor.

We traverse and process the new vnode:

First find the corresponding node from the old vnode array. If it is found, it means it can be reused. Then just move it.

If not found, insert it and the anchor point is the nextSibling of the previous node.

那如果找到了可复用的节点之后，那移动到哪里呢？

其实新的 vnode 数组中记录的顺序就是目标的顺序。所以把对应的节点按照新 vnode 数组的顺序来移动就好了。

const prevVNode = newChildren[i - 1]
if (prevVNode) {
    const anchor = prevVNode.el.nextSibling
    insert(newVNode.el, container, anchor)
}

要插入到 i 的位置，那就要取 i-1 位置的节点的 nextSibling 做为锚点来插入当前节点。

但是并不是所有的节点都需要移动，比如处理到第二个新的 vnode，发现它在旧的 vnode 数组中的下标为 4，说明本来就是在后面了，那就不需要移动了。反之，如果是 vnode 查找到的对应的旧的 vnode 在当前 index 之前才需要移动。

也就是这样：

let j = 0
let find = false
// 遍历旧的 children
for (j; j < oldChildren.length; j++) {
    const oldVNode = oldChildren[j]
    // 如果找到了具有相同 key 值的两个节点，则调用 patch 函数更新之
    if (newVNode.key === oldVNode.key) {
        find = true
        patch(oldVNode, newVNode, container)

        if (j < lastIndex) { // 旧的 vnode 数组的下标在上一个 index 之前，需要移动
          const prevVNode = newChildren[i - 1]
          if (prevVNode) {
            const anchor = prevVNode.el.nextSibling
            insert(newVNode.el, container, anchor)
          }
        } else {// 不需要移动
          // 更新 lastIndex
          lastIndex = j
        }
        break
    }
}

查找新的 vnode 在旧的 vnode 数组中的下标，如果找到了的话，说明对应的 dom 就是可以复用的，先 patch 一下，然后移动。

移动的话判断下下标是否在 lastIndex 之后，如果本来就在后面，那就不用移动，更新下 lastIndex 就行。

如果下标在 lastIndex 之前，说明需要移动，移动到的位置前面分析过了，就是就是新 vnode 数组 i-1 的后面。

这样，我们就完成了 dom 节点的复用和移动。

新的 vnode 数组全部处理完后，旧的 vnode 数组可能还剩下一些不再需要的，那就删除它们：

// 遍历旧的节点
for (let i = 0; i < oldChildren.length; i++) {
    const oldVNode = oldChildren[i]
    // 拿着旧 VNode 去新 children 中寻找相同的节点
    const has = newChildren.find(
      vnode => vnode.key === oldVNode.key
    )
    if (!has) {
      // 如果没有找到相同的节点，则移除
      unmount(oldVNode)
    }
}

这样，我们就完成了两组 vnode 的 diff 和对应 dom 的增删改。

小结一下：

diff 算法的目的是根据 key 复用 dom 节点，通过移动节点而不是创建新节点来减少 dom 操作。

对于每个新的 vnode，在旧的 vnode 中根据 key 查找一下，如果没查找到，那就新增 dom 节点，如果查找到了，那就可以复用。

复用的话要不要移动要判断下下标，如果下标在 lastIndex 之后，就不需要移动，因为本来就在后面，反之就需要移动。

最后，把旧的 vnode 中在新 vnode 中没有的节点从 dom 树中删除。

这就是一个完整的 diff 算法的实现。

这个 diff 算法我们是从一端逐个处理的，叫做简单 diff 算法。

简单 diff 算法其实性能不是最好的，比如旧的 vnode 数组是 ABCD，新的 vnode 数组是 DABC，按照简单 diff 算法，A、B、C 都需要移动。

那怎么优化这个算法呢？

从一个方向顺序处理会有这个问题，那从两个方向同时对比呢？

这就是双端 diff 算法：

双端 diff

简单 diff 算法能够实现 dom 节点的复用，但有的时候会做一些没必要的移动。双端 diff 算法解决了这个问题，它是从两端进行对比。

我们需要 4 个指针，分别指向新旧两个 vnode 数组的头尾：

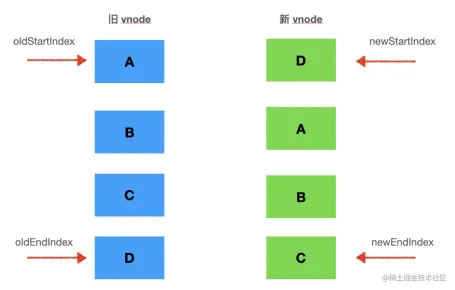
头和尾的指针向中间移动，直到 oldStartIdx

每次对比下两个头指针指向的节点、两个尾指针指向的节点，头和尾指向的节点，是不是 key是一样的，也就是可复用的。

如果是可复用的话就直接用，调用 patch 更新一下，如果是头尾这种，还要移动下位置。

也就是这样的：

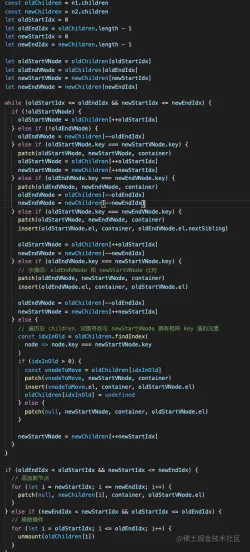
while (oldStartIdx <= oldEndIdx && newStartIdx <= newEndIdx) {
  if (oldStartVNode.key === newStartVNode.key) { // 头头
    patch(oldStartVNode, newStartVNode, container)
    oldStartVNode = oldChildren[++oldStartIdx]
    newStartVNode = newChildren[++newStartIdx]
  } else if (oldEndVNode.key === newEndVNode.key) {//尾尾
    patch(oldEndVNode, newEndVNode, container)
    oldEndVNode = oldChildren[--oldEndIdx]
    newEndVNode = newChildren[--newEndIdx]
  } else if (oldStartVNode.key === newEndVNode.key) {//头尾，需要移动
    patch(oldStartVNode, newEndVNode, container)
    insert(oldStartVNode.el, container, oldEndVNode.el.nextSibling)

    oldStartVNode = oldChildren[++oldStartIdx]
    newEndVNode = newChildren[--newEndIdx]
  } else if (oldEndVNode.key === newStartVNode.key) {//尾头，需要移动
    patch(oldEndVNode, newStartVNode, container)
    insert(oldEndVNode.el, container, oldStartVNode.el)

    oldEndVNode = oldChildren[--oldEndIdx]
    newStartVNode = newChildren[++newStartIdx]
  } else {
    
    // 头尾没有找到可复用的节点
  }
}

头头和尾尾的对比比较简单，头尾和尾头的对比还要移动下节点。

比如旧 vnode 的头节点是新的 vnode 的尾节点，那就要把它移动到旧的 vnode 的尾节点的位置。

也就是：

insert(oldStartVNode.el, container, oldEndVNode.el.nextSibling)

插入节点的锚点节点是 oldEndVNode 对应的 dom 节点的 nextSibling。

如果旧 vnode 的尾节点是新 vnode 的头结点，那就要把它移动到旧 vnode 的头结点的位置。

也就是：

insert(oldEndVNode.el, container, oldStartVNode.el)

插入节点的锚点节点是 oldStartVNode 对应的 dom 节点（因为要插在它之前）。

从双端进行对比，能尽可能的减少节点移动的次数。

当然，还要处理下如果双端都没有可复用节点的情况：

如果双端都没有可复用节点，那就在旧节点数组中找，找到了就把它移动过来，并且原位置置为 undefined。没找到的话就插入一个新的节点。

也就是这样：

const idxInOld = oldChildren.findIndex(
  node => node.key === newStartVNode.key
)
if (idxInOld > 0) {
  const vnodeToMove = oldChildren[idxInOld]
  patch(vnodeToMove, newStartVNode, container)
  insert(vnodeToMove.el, container, oldStartVNode.el)
  oldChildren[idxInOld] = undefined
} else {
  patch(null, newStartVNode, container, oldStartVNode.el)
}

因为有了一些 undefined 的节点，所以要加上空节点的处理逻辑：

if (!oldStartVNode) {
    oldStartVNode = oldChildren[++oldStartIdx]
} else if (!oldEndVNode) {
    oldEndVNode = newChildren[--oldEndIdx]
}

这样就完成了节点的复用和移动的逻辑。

那确实没有可复用的节点的那些节点呢？

经过前面的移动之后，剩下的节点都被移动到了中间，如果新 vnode 有剩余，那就批量的新增，如果旧 vnode 有剩余那就批量的删除。

因为前面一个循环的判断条件是 oldStartIdx

所以判断条件是这样的：

if (oldEndIdx < oldStartIdx && newStartIdx <= newEndIdx) {
  // 添加新节点
  for (let i = newStartIdx; i <= newEndIdx; i++) {
    patch(null, newChildren[i], container, oldStartVNode.el)
  }
} else if (newEndIdx < newStartIdx && oldStartIdx <= oldEndIdx) {
  // 移除操作
  for (let i = oldStartIdx; i <= oldEndIdx; i++) {
    unmount(oldChildren[i])
  }
}

这样就是一个完整的 diff 算法了，包括查找可复用节点和移动节点、新增和删除节点。

而且因为从两侧查找节点，会比简单 diff 算法性能更好一些。

比如 ABCD 到 DABC，简单 diff 算法需要移动 ABC 三个节点，而双端 diff 算法只需要移动 D 一个节点。

小结一下：

双端 diff 是头尾指针向中间移动的同时，对比头头、尾尾、头尾、尾头是否可以复用，如果可以的话就移动对应的 dom 节点。

如果头尾没找到可复用节点就遍历 vnode 数组来查找，然后移动对应下标的节点到头部。

最后还剩下旧的 vnode 就批量删除，剩下新的 vnode 就批量新增。

双端 diff 算法是 Vue2 采用的 diff 算法，性能还不错。

后来，Vue3 又对 diff 算法进行了一次升级，叫做快速 diff 算法。这个后面再讲。

总结

React 和 Vue 都是基于 vdom 的前端框架，组件产生 vdom，渲染器再把 vdom 通过增删改的 dom api 更新到 dom。

当再次渲染出 vdom 时，就要新旧两棵 vdom 树做 diff，只更新变化的 dom 节点。

两棵树的 diff 是 O(n^3) 的，时间复杂度太高，因此前端框架规定了只做同层 diff，还有 type 不一样就认为节点不一样，不再对比子节点。这样时间复杂度一下子就降到了 O(n)。

但是对于多个子字节点的 diff 不能粗暴的删除和新增，要尽量复用已有的节点，也就是通过移动代替新增。

所以多节点的时候，要指定 key，然后 diff 算法根据 key 来查找和复用节点。

简单 diff 算法是依次根据 key 查找旧节点的，移动的话通过 lastIndex 判断，大于它就不用动，小于它才需要移动。剩下的节点再批量删除和新增。

但是简单 diff 算法局限性还是比较大的，有些情况下性能并不好，所以 vue2 用的是双端 diff 算法。

双端 diff 算法是头尾指针向中间移动，分别判断头尾节点是否可以复用，如果没有找到可复用的节点再去遍历查找对应节点的下标，然后移动。全部处理完之后也要对剩下的节点进行批量的新增和删除。

其实 diff 算法最重要的就是找到可复用的节点，然后移动到正确的位置。只不过不同的算法查找顺序不一样。

vue2 是用的双端 diff 的算法，而 vue3 则通过最长递增子序列的算法做了进一步的优化，关于优化后的 diff 算法，我们之后再聊。

【相关视频教程推荐：web前端】

The above is the detailed content of Learn more about the double-ended diff algorithm in Vue. For more information, please follow other related articles on the PHP Chinese website!