Minimum Spanning Tree-Prim's Algorithm and Kruskal's Algorithm
The spanning tree of a graph is an acyclic connected subgraph containing all vertices, a weighted graph The minimum spanning tree of is its spanning tree with the smallest weight.
Prim algorithm
Simple description of the algorithm
1). Input: a weighted connected graph, in which the vertex set is V and the edge set is E;
2). Initialization: Vnew = {x}, where x is any node (starting point) in the set V, Enew = {}, is empty;
3). Repeat the following Operation until Vnew = V:
a. Select the edge with the smallest weight in set E , where u is the set V elements in new, and v is not in the Vnew set, and v∈V (if there are multiple edges that meet the above conditions and have the same weight, you can choose arbitrarily One of them);
b. Add v to the set Vnew, and add the edge to the set Enew Medium;
4). Output: Use the set Vnew and Enew to describe the resulting minimum spanning tree.
The following is an illustration description of the algorithm
| Legend | Description | Not optional | Optional | Selected(Vnew) |
|---|---|---|---|---|
|
|
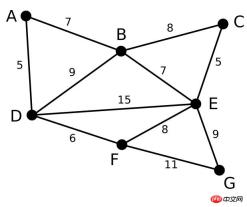
This is the original weighted connected graph. The number on one side of each edge represents its weight. | - | - | ##- |
|
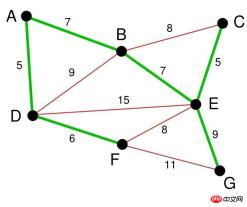
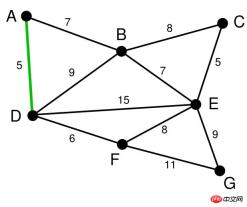
Vertex D is arbitrarily chosen as the starting point. Vertices A, B, E, and F are connected to D by a single edge. A is the vertex closest to D, so A and the corresponding edge AD are highlighted. | C, G | A, B, E, F | D |
|
| ##The next vertex is distanceD Or A nearest vertex. B is 9 from D, 7 from A, 15 from E, and 6 from F. Therefore, F is closest to D or A, so the vertex F and the corresponding edge DF are highlighted. | C, GB, E, F | A, D | |
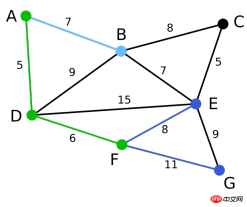 | The algorithm continues to repeat the above steps. Vertex B with a distance of 7 from A is highlighted. | CB, E, G | A, D, F | |
|
|
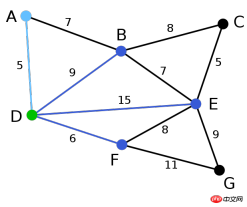
In the current situation, you can choose between C, E and G. C is 8 from B, E is 7 from B, and G is 11 from F. E is closest, so the vertex E and the corresponding edge BE are highlighted. | None | C, E, G | A, D, F, B |
|
|
##Here, the only vertices to choose from are C and G. The distance between C and E is 5, and the distance between G and E is 9, so C is selected and combined with the edge EC are highlighted together. | None | C, G | ##A, D, F, B, E|
|
| Gis the only remaining vertex, Its distance from F is 11, its distance from E is 9, and E is the closest, so G and the corresponding edge EG are highlighted. None | G | ##A, D, F, B, E, C | |
|
None | None | ##A, D, F, B, E, C, G | For algorithm implementation, please refer to the fourth edition of "Algorithm", or "Data Structure - Java Language Implementation" by Tsinghua Publishing House (the implementation method is clearer and simpler)
Kruskal Algorithm 1. Overview Kruskal's algorithm is an algorithm used to find the minimum spanning tree, published by Joseph Kruskal in 1956. There are also Prim algorithm and Boruvka algorithm used to solve the same problem. All three algorithms are applications of greedy algorithms. The difference from Boruvka's algorithm is that Kruskal's algorithm is also effective when there are edges with the same weight in the graph.
2. Brief description of the algorithm 1). Remember that there are v vertices and e edges in Graph 2). Create a new graph Graphnew, and Graphnew has the original graph The same e vertices, but no edges 3). Sort all e edges in the original graph Graph from small to large by weight 4). Loop: start from the edge with the smallest weight and traverse each edge until all nodes in the graph are in the same connected component ## If this edge is connected The two nodes in the graph Graphnew are not in the same connected component. Legend description:First of all, we have a graph Graph with several points and edges Sort the lengths of all edges, and use the sorted results as the basis for our edge selection. Here again the idea of greedy algorithm is reflected.
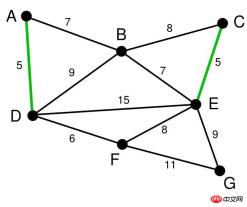
Look for the remaining changes. We found CE. The weight here is also 5 and so on. We find 6, 7, 7, that is, DF, AB, BE.
In the end, only EG and FG are left. Of course we chose EG. Algorithm implementation can refer to the code in the fourth edition of "Algorithm".
|
The above is the detailed content of Detailed explanation of examples of minimum spanning trees. For more information, please follow other related articles on the PHP Chinese website!
 Is Java Platform Independent if then how?May 09, 2025 am 12:11 AM
Is Java Platform Independent if then how?May 09, 2025 am 12:11 AMJava is platform-independent because of its "write once, run everywhere" design philosophy, which relies on Java virtual machines (JVMs) and bytecode. 1) Java code is compiled into bytecode, interpreted by the JVM or compiled on the fly locally. 2) Pay attention to library dependencies, performance differences and environment configuration. 3) Using standard libraries, cross-platform testing and version management is the best practice to ensure platform independence.
 The Truth About Java's Platform Independence: Is It Really That Simple?May 09, 2025 am 12:10 AM
The Truth About Java's Platform Independence: Is It Really That Simple?May 09, 2025 am 12:10 AMJava'splatformindependenceisnotsimple;itinvolvescomplexities.1)JVMcompatibilitymustbeensuredacrossplatforms.2)Nativelibrariesandsystemcallsneedcarefulhandling.3)Dependenciesandlibrariesrequirecross-platformcompatibility.4)Performanceoptimizationacros
 Java Platform Independence: Advantages for web applicationsMay 09, 2025 am 12:08 AM
Java Platform Independence: Advantages for web applicationsMay 09, 2025 am 12:08 AMJava'splatformindependencebenefitswebapplicationsbyallowingcodetorunonanysystemwithaJVM,simplifyingdeploymentandscaling.Itenables:1)easydeploymentacrossdifferentservers,2)seamlessscalingacrosscloudplatforms,and3)consistentdevelopmenttodeploymentproce
 JVM Explained: A Comprehensive Guide to the Java Virtual MachineMay 09, 2025 am 12:04 AM
JVM Explained: A Comprehensive Guide to the Java Virtual MachineMay 09, 2025 am 12:04 AMTheJVMistheruntimeenvironmentforexecutingJavabytecode,crucialforJava's"writeonce,runanywhere"capability.Itmanagesmemory,executesthreads,andensuressecurity,makingitessentialforJavadeveloperstounderstandforefficientandrobustapplicationdevelop
 Key Features of Java: Why It Remains a Top Programming LanguageMay 09, 2025 am 12:04 AM
Key Features of Java: Why It Remains a Top Programming LanguageMay 09, 2025 am 12:04 AMJavaremainsatopchoicefordevelopersduetoitsplatformindependence,object-orienteddesign,strongtyping,automaticmemorymanagement,andcomprehensivestandardlibrary.ThesefeaturesmakeJavaversatileandpowerful,suitableforawiderangeofapplications,despitesomechall
 Java Platform Independence: What does it mean for developers?May 08, 2025 am 12:27 AM
Java Platform Independence: What does it mean for developers?May 08, 2025 am 12:27 AMJava'splatformindependencemeansdeveloperscanwritecodeonceandrunitonanydevicewithoutrecompiling.ThisisachievedthroughtheJavaVirtualMachine(JVM),whichtranslatesbytecodeintomachine-specificinstructions,allowinguniversalcompatibilityacrossplatforms.Howev
 How to set up JVM for first usage?May 08, 2025 am 12:21 AM
How to set up JVM for first usage?May 08, 2025 am 12:21 AMTo set up the JVM, you need to follow the following steps: 1) Download and install the JDK, 2) Set environment variables, 3) Verify the installation, 4) Set the IDE, 5) Test the runner program. Setting up a JVM is not just about making it work, it also involves optimizing memory allocation, garbage collection, performance tuning, and error handling to ensure optimal operation.
 How can I check Java platform independence for my product?May 08, 2025 am 12:12 AM
How can I check Java platform independence for my product?May 08, 2025 am 12:12 AMToensureJavaplatformindependence,followthesesteps:1)CompileandrunyourapplicationonmultipleplatformsusingdifferentOSandJVMversions.2)UtilizeCI/CDpipelineslikeJenkinsorGitHubActionsforautomatedcross-platformtesting.3)Usecross-platformtestingframeworkss


Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

Dreamweaver Mac version
Visual web development tools

SAP NetWeaver Server Adapter for Eclipse
Integrate Eclipse with SAP NetWeaver application server.

SublimeText3 Chinese version
Chinese version, very easy to use

MantisBT
Mantis is an easy-to-deploy web-based defect tracking tool designed to aid in product defect tracking. It requires PHP, MySQL and a web server. Check out our demo and hosting services.

DVWA
Damn Vulnerable Web App (DVWA) is a PHP/MySQL web application that is very vulnerable. Its main goals are to be an aid for security professionals to test their skills and tools in a legal environment, to help web developers better understand the process of securing web applications, and to help teachers/students teach/learn in a classroom environment Web application security. The goal of DVWA is to practice some of the most common web vulnerabilities through a simple and straightforward interface, with varying degrees of difficulty. Please note that this software





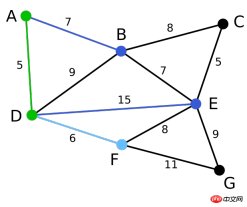
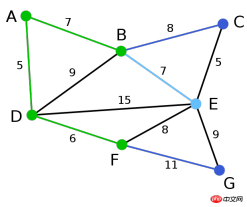
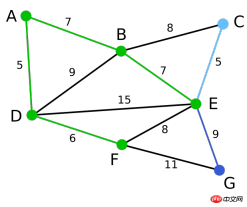
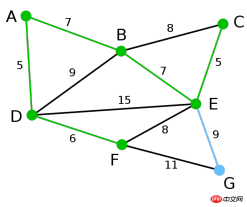
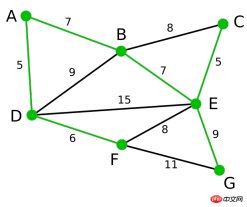
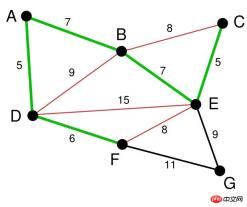 Continue to select BC or EF although the side with a length of 8 is now the smallest unselected side. But now they are connected (BC can be connected through CE, EB,
Continue to select BC or EF although the side with a length of 8 is now the smallest unselected side. But now they are connected (BC can be connected through CE, EB,