Python zeichnet 3D-Grafiken

Dieser Artikel stellt hauptsächlich Python zum Zeichnen von 3D-Grafiken vor, was einen gewissen Referenzwert hat. Jetzt kann ich ihn mit allen teilen, die ihn brauchen.

3D-Grafiken werden in der Datenanalyse und Datenmodellierung verwendet. Es wird häufig in Bereichen wie Bildverarbeitung und Bildverarbeitung verwendet. Im Folgenden werde ich Ihnen vorstellen, wie Sie mit Python 3D-Grafiken zeichnen, einschließlich 3D-Streuungspunkten, 3D-Oberflächen, 3D-Konturen und geraden 3D-Linien. Kurven) und 3D-Text.

Vorbereitungsarbeit:

Um 3D-Grafiken in Python zu zeichnen, verwenden Sie weiterhin das häufig verwendete Zeichenmodul matplotlib, müssen jedoch das mpl_toolkits-Toolkit installieren. Die Installationsmethode lautet wie folgt: Geben Sie das ein Python-Installationsverzeichnis über die Windows-Befehlszeile Führen Sie im Ordner „Skripte“ Folgendes aus: pip install --upgrade matplotlib; Führen Sie diesen Befehl direkt in der Linux-Umgebung aus.

Nach der Installation dieses Moduls können Sie die Klasse mplot3d unter mpl_tookits aufrufen, um 3D-Grafiken zu zeichnen.

Das Folgende ist ein Beispiel.

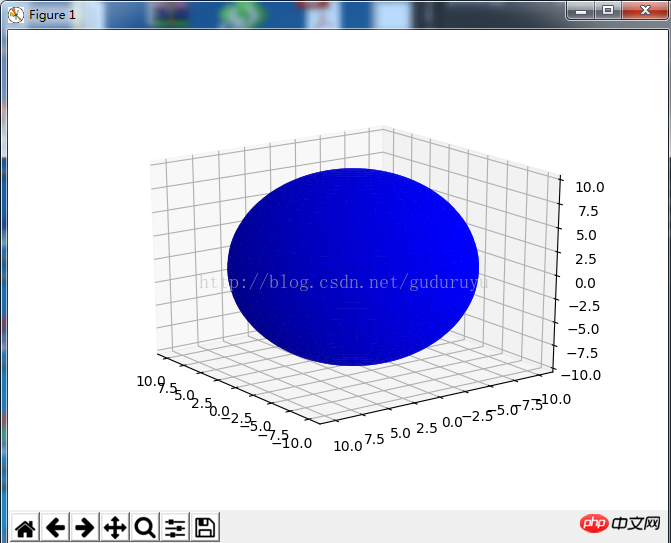
1. Zeichnung der 3D-Oberflächenform

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
import numpy as np 
 
fig = plt.figure() 
ax = fig.add_subplot(111, projection='3d') 
 
# Make data 
u = np.linspace(0, 2 * np.pi, 100) 
v = np.linspace(0, np.pi, 100) 
x = 10 * np.outer(np.cos(u), np.sin(v)) 
y = 10 * np.outer(np.sin(u), np.sin(v)) 
z = 10 * np.outer(np.ones(np.size(u)), np.cos(v)) 
 
# Plot the surface 
ax.plot_surface(x, y, z, color='b') 
 
plt.show()

Kugelförmige Oberfläche, die Ergebnisse sind wie folgt:

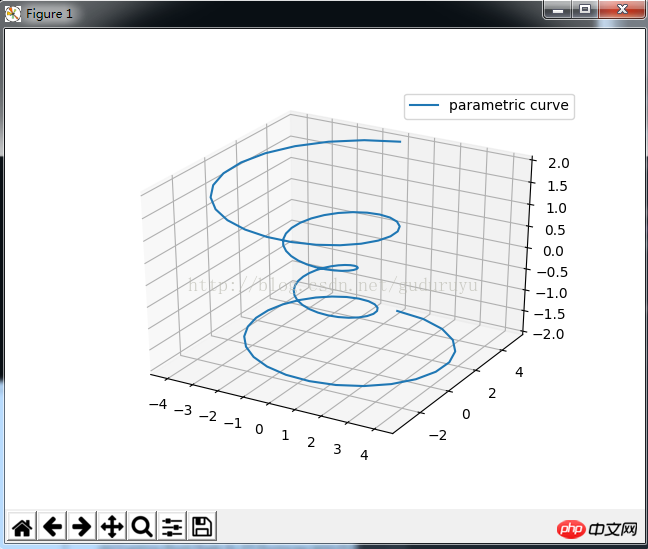
2. Zeichnen von 3D-Geraden (Kurven)

import matplotlib as mpl 
from mpl_toolkits.mplot3d import Axes3D 
import numpy as np 
import matplotlib.pyplot as plt 
 
mpl.rcParams['legend.fontsize'] = 10 
 
fig = plt.figure() 
ax = fig.gca(projection='3d') 
theta = np.linspace(-4 * np.pi, 4 * np.pi, 100) 
z = np.linspace(-2, 2, 100) 
r = z**2 + 1 
x = r * np.sin(theta) 
y = r * np.cos(theta) 
ax.plot(x, y, z, label='parametric curve') 
ax.legend() 
 
plt.show()

Dieser Code wird zum Zeichnen einer spiralförmigen 3D-Kurve verwendet Die Ergebnisse sind wie folgt:

3D-Konturen zeichnen

from mpl_toolkits.mplot3d import axes3d 
import matplotlib.pyplot as plt 
from matplotlib import cm 
 
fig = plt.figure() 
ax = fig.gca(projection='3d') 
X, Y, Z = axes3d.get_test_data(0.05) 
cset = ax.contour(X, Y, Z, zdir='z', offset=-100, cmap=cm.coolwarm) 
cset = ax.contour(X, Y, Z, zdir='x', offset=-40, cmap=cm.coolwarm) 
cset = ax.contour(X, Y, Z, zdir='y', offset=40, cmap=cm.coolwarm) 
 
ax.set_xlabel('X') 
ax.set_xlim(-40, 40) 
ax.set_ylabel('Y') 
ax.set_ylim(-40, 40) 
ax.set_zlabel('Z') 
ax.set_zlim(-100, 100) 
 
plt.show()

Die Zeichenergebnisse sind wie folgt:

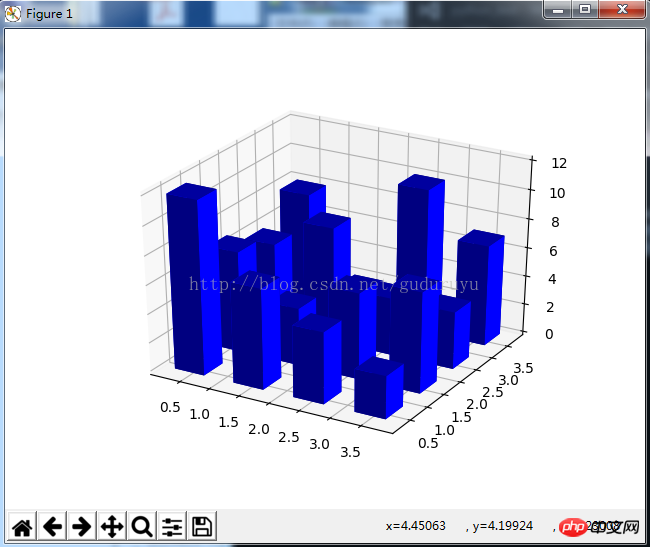
3D-Histogramm zeichnen

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
import numpy as np 
 
fig = plt.figure() 
ax = fig.add_subplot(111, projection='3d') 
x, y = np.random.rand(2, 100) * 4 
hist, xedges, yedges = np.histogram2d(x, y, bins=4, range=[[0, 4], [0, 4]]) 
 
# Construct arrays for the anchor positions of the 16 bars. 
# Note: np.meshgrid gives arrays in (ny, nx) so we use 'F' to flatten xpos, 
# ypos in column-major order. For numpy >= 1.7, we could instead call meshgrid 
# with indexing='ij'. 
xpos, ypos = np.meshgrid(xedges[:-1] + 0.25, yedges[:-1] + 0.25) 
xpos = xpos.flatten('F') 
ypos = ypos.flatten('F') 
zpos = np.zeros_like(xpos) 
 
# Construct arrays with the dimensions for the 16 bars. 
dx = 0.5 * np.ones_like(zpos) 
dy = dx.copy() 
dz = hist.flatten() 
 
ax.bar3d(xpos, ypos, zpos, dx, dy, dz, color='b', zsort='average') 
 
plt.show()

Die Zeichenergebnisse sind wie folgt:

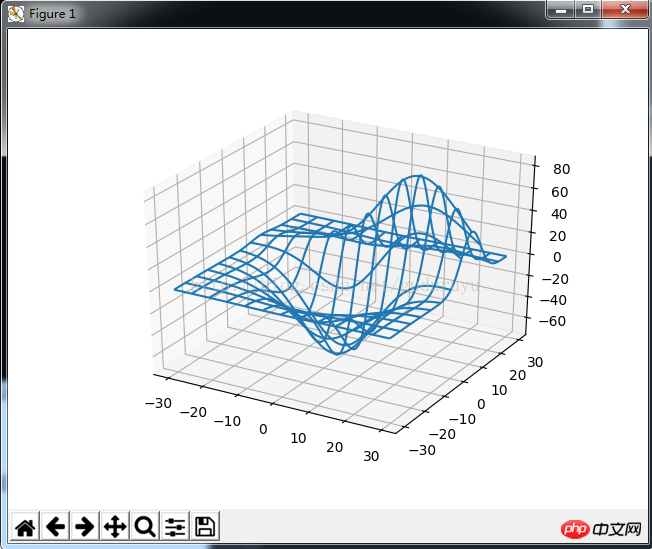
3D-Netzlinien zeichnen

from mpl_toolkits.mplot3d import axes3d 
import matplotlib.pyplot as plt 
 
 
fig = plt.figure() 
ax = fig.add_subplot(111, projection='3d') 
 
# Grab some test data. 
X, Y, Z = axes3d.get_test_data(0.05) 
 
# Plot a basic wireframe. 
ax.plot_wireframe(X, Y, Z, rstride=10, cstride=10) 
 
plt.show()

Die Zeichenergebnisse sind wie folgt:

3D-Dreieck-Patch-Diagramm zeichnen

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
import numpy as np 
 
 
n_radii = 8 
n_angles = 36 
 
# Make radii and angles spaces (radius r=0 omitted to eliminate duplication). 
radii = np.linspace(0.125, 1.0, n_radii) 
angles = np.linspace(0, 2*np.pi, n_angles, endpoint=False) 
 
# Repeat all angles for each radius. 
angles = np.repeat(angles[..., np.newaxis], n_radii, axis=1) 
 
# Convert polar (radii, angles) coords to cartesian (x, y) coords. 
# (0, 0) is manually added at this stage, so there will be no duplicate 
# points in the (x, y) plane. 
x = np.append(0, (radii*np.cos(angles)).flatten()) 
y = np.append(0, (radii*np.sin(angles)).flatten()) 
 
# Compute z to make the pringle surface. 
z = np.sin(-x*y) 
 
fig = plt.figure() 
ax = fig.gca(projection='3d') 
 
ax.plot_trisurf(x, y, z, linewidth=0.2, antialiased=True) 
 
plt.show(

Die Zeichnungsergebnisse sind wie folgt:

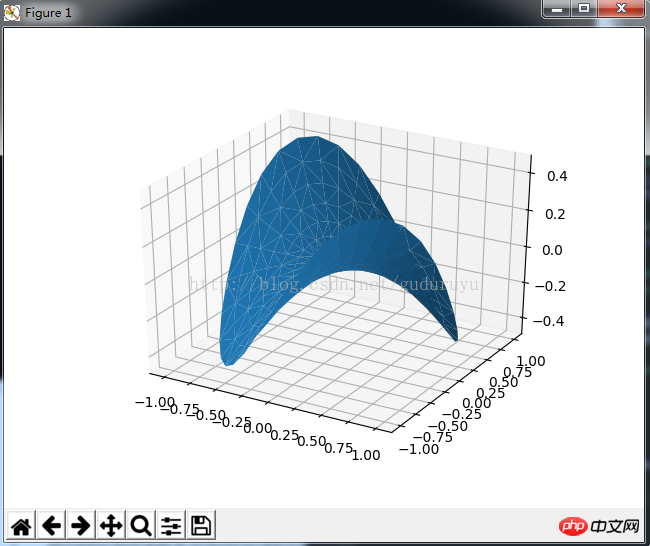
7. Zeichnen Sie ein 3D-Streudiagramm

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
import numpy as np 
 
 
def randrange(n, vmin, vmax): 
 ''''' 
 Helper function to make an array of random numbers having shape (n, ) 
 with each number distributed Uniform(vmin, vmax). 
 ''' 
 return (vmax - vmin)*np.random.rand(n) + vmin 
 
fig = plt.figure() 
ax = fig.add_subplot(111, projection='3d') 
 
n = 100 
 
# For each set of style and range settings, plot n random points in the box 
# defined by x in [23, 32], y in [0, 100], z in [zlow, zhigh]. 
for c, m, zlow, zhigh in [('r', 'o', -50, -25), ('b', '^', -30, -5)]: 
 xs = randrange(n, 23, 32) 
 ys = randrange(n, 0, 100) 
 zs = randrange(n, zlow, zhigh) 
 ax.scatter(xs, ys, zs, c=c, marker=m) 
 
ax.set_xlabel('X Label') 
ax.set_ylabel('Y Label') 
ax.set_zlabel('Z Label') 
 
plt.show()

Die Zeichnung Die Ergebnisse lauten wie folgt:

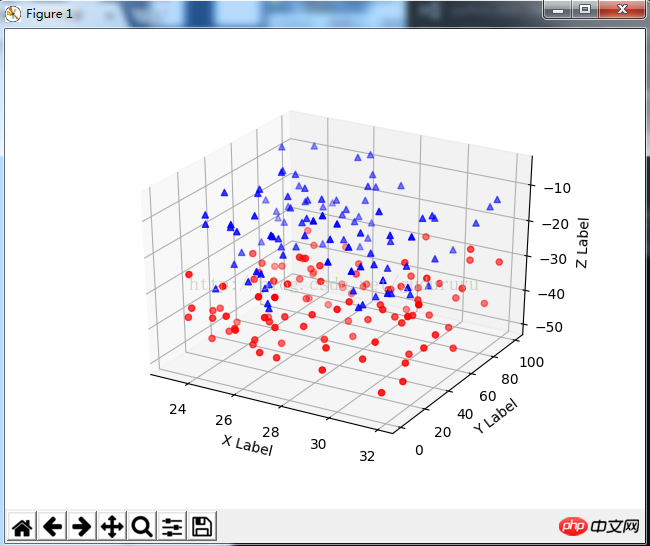
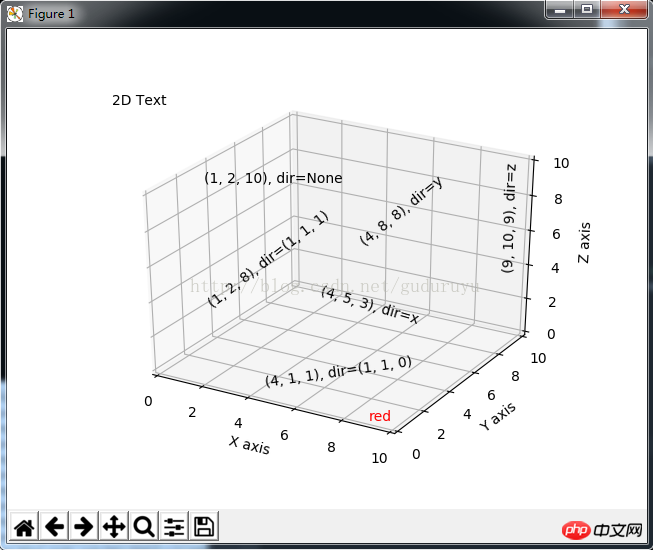
8. 3D-Text zeichnen

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
 
 
fig = plt.figure() 
ax = fig.gca(projection='3d') 
 
# Demo 1: zdir 
zdirs = (None, 'x', 'y', 'z', (1, 1, 0), (1, 1, 1)) 
xs = (1, 4, 4, 9, 4, 1) 
ys = (2, 5, 8, 10, 1, 2) 
zs = (10, 3, 8, 9, 1, 8) 
 
for zdir, x, y, z in zip(zdirs, xs, ys, zs): 
 label = '(%d, %d, %d), dir=%s' % (x, y, z, zdir) 
 ax.text(x, y, z, label, zdir) 
 
# Demo 2: color 
ax.text(9, 0, 0, "red", color='red') 
 
# Demo 3: text2D 
# Placement 0, 0 would be the bottom left, 1, 1 would be the top right. 
ax.text2D(0.05, 0.95, "2D Text", transform=ax.transAxes) 
 
# Tweaking display region and labels 
ax.set_xlim(0, 10) 
ax.set_ylim(0, 10) 
ax.set_zlim(0, 10) 
ax.set_xlabel('X axis') 
ax.set_ylabel('Y axis') 
ax.set_zlabel('Z axis') 
 
plt.show(

Die Zeichnungsergebnisse sind wie folgt:

9. 3D-Balkendiagramm

from mpl_toolkits.mplot3d import Axes3D 
import matplotlib.pyplot as plt 
import numpy as np 
 
fig = plt.figure() 
ax = fig.add_subplot(111, projection='3d') 
for c, z in zip(['r', 'g', 'b', 'y'], [30, 20, 10, 0]): 
 xs = np.arange(20) 
 ys = np.random.rand(20) 
 
 # You can provide either a single color or an array. To demonstrate this, 
 # the first bar of each set will be colored cyan. 
 cs = [c] * len(xs) 
 cs[0] = 'c' 
 ax.bar(xs, ys, zs=z, zdir='y', color=cs, alpha=0.8) 
 
ax.set_xlabel('X') 
ax.set_ylabel('Y') 
ax.set_zlabel('Z') 
 
plt.show()

Das Die Zeichenergebnisse lauten wie folgt:

Verwandte Empfehlungen:

Verwenden Sie Python, um häufig verwendete Diagramme zu zeichnen

Detaillierte Beispiele zum Zeichnen von Grafiken mit Python

Das obige ist der detaillierte Inhalt vonPython zeichnet 3D-Grafiken. Für weitere Informationen folgen Sie bitte anderen verwandten Artikeln auf der PHP chinesischen Website!